Topological properties of two-dimensional number systems

J

OURNAL DE

T

HÉORIE DES

N

OMBRES DE

B

ORDEAUX

S

HIGEKI

A

KIYAMA

J

ÖRG

M. T

HUSWALDNER

Topological properties of two-dimensional number systems

Journal de Théorie des Nombres de Bordeaux, tome

12, n

o

_{1 (2000),}

p. 69-79

<http://www.numdam.org/item?id=JTNB_2000__12_1_69_0>

© Université Bordeaux 1, 2000, tous droits réservés.

L’accès aux archives de la revue « Journal de Théorie des Nombres de Bordeaux » (http://jtnb.cedram.org/) implique l’accord avec les condi-tions générales d’utilisation (http://www.numdam.org/conditions). Toute uti-lisation commerciale ou impression systématique est constitutive d’une infraction pénale. Toute copie ou impression de ce fichier doit conte-nir la présente mention de copyright.

Article numérisé dans le cadre du programme Numérisation de documents anciens mathématiques

,

69-Topological Properties

of Two-Dimensional

Number

_Systems

par SHIGEKI AKIYAMA et JÖRG M. THUSWALDNER

RÉSUMÉ. Pour une matrice réelle M d’ordre 2

_donnée,

on _peut

définir la notion de

_{représentation M-adique}

d’un élément de ]R2.

On note F le domaine fondamental constitué des nombres de R2

dont le

_{développement "M-adique"}

ne commence _{pas par} 0. C’est

l’analogue

dans R2 des nombres

_q-adiques,

où la matrice M

_joue

le rôle de la base _q. Kátai et

_Környei

ont démontré

_{que F}

est

com-pact, et que R2 s’écrit comme la réunion dénombrable de certains

translatés de

_F,

l’intersection de 2

_quelconques

d’entre eux étant

de mesure nulle. Dans cet

article,

nous construisons des

_points

qui

appartiennent

simultanément à trois translatés de _F, et nous

montrons

_{que F}

est connexe. Nous donnons aussi une

_propriété

sur la structure des

_points

intérieurs de F.

ABSTRACT. In the two dimensional real vector space R2 one can

define

_analogs

of the well-known

_q-adic

number _systems. In these

number _systems a matrix M

plays

the role of the base number _q.

In the _{present paper} we

_study

the so-called fundamental domain

F of such number _systems. This is the set of all elements of R2

having

zero

_integer

_part in their "M-adic" _{representation.} It was

proved by

Kátai and

_Környei,

that F is a _compact set and certain

translates of it form a

_tiling

of the R2. We construct _points, where three different tiles of this

_tiling

coincide.

_Furthermore,

we _prove

the connectedness of F and

_give

a result on the structure of its inner _points.

1. INTRODUCTION

In this _paper we use the

_following

notations:

_R,

Q,

Z and N denote

the set of real

_numbers,

rational

_numbers,

_integers

and

_positive

_integers,

respectively.

If x e R we will write

Lx J

for the

largest integer

less than or

equal

to x. _{A will denote the 2-dimensional}

_Lebesgue

measure.

Further-more, we write 8A for the

_boundary

of the set A and

_int(A)

for its interior.

diag(al, a2)

denotes a 2 x 2

_diagonal

matrix with

_diagonal

elements

À1

and

À2.

Let

_{q &#x3E;}

2 be an

_integer.

Then each

_{positive integer n}

has a

unique

q-adic

_{representation}

of the

_{akq k}

with _{ak E}

_{{0,1, ... ,}

q -

1}

(0

k

_H)

and 0 for 0. These

_q-adic

number

_systems

have been

_generalized

in various ways. In the

present

paper we deal with

analogs

of these number

_systems

in the 2-dimensional real _{vector space,} that _emerge from number

_systems

in

_quadratic

number fields. The first

major

step

in the

_{investigation}

of number

_systems

in number fields was

done

_by

Knuth

_[13],

who studied number

_systems

with

_negative

bases as

well as number

_systems

in the

_ring

of Gaussian

_{integers. Meanwhile, Katai,}

Kovacs,

Peth6 and Szab6 invented a

general

notion of number

_systems

in

rings

of

_integers

of number

_fields,

the so-called canonical number

_systems

(cf.

for instance

_[10,

_{11, 12,}

_15]).

We recall their definition.

Let K be a number field with

_ring

of

_integers

_ZK.

For an

algebraic

integer

b E

_ZK

define

N

=

{O, 1,... , IN(b)1 - 1},

where

N(b)

denotes the

norm of b over

Q.

The

pair

(b, N)

is called a canonical number

_system

if

any y E

ZK

admits a

_{representation}

of the

_shape

where ck

E JV

(0

k

H)

and 0 for 0.

These number

_systems

resemble a natural

generalization

of

q-adic

num-ber

_systems

to number fields. Each of these number

_systems

_gives

rise to

a number

_system

in the n-dimensional real vector _space. Since we are

only

interested in the 2-dimensional case, we construct these number

_systems

only

for this case. Consider a canonical number

_system

in a

qua-dratic number field K with

_ring

of

_{integers ZK.}

Let + Ax + B

be the minimal

_polynomial

of b. It is

_known,

that for bases of canonical number

_systems

-1 A B &#x3E; 2 holds

_(cf.

_[10,

_11,

_12]).

Now consider the

_{embedding 4P :}

K -

_Jae2,

al +

_a2b

H

_(al,

a2),

where _a,, a2 E

_Q.

Ko-vacs

[14]

_proved,

that

{1,

b}

forms an

integral

basis of

ZK.

Thus we have

4l(ZK)

=

Z2 . Furthermore,

_note that = with

Since the elements

of .N

are rational

integers,

for each c E

_{N, 4l (c) = (c,}

0)T .

Summing

up we _see, that

(M,

4l(N))

forms a number

_system

in the two

dimensional real vector _space in the

_following

sense

(cf.

also

[8],

where some

_properties

of these number

_systems

are

studied).

Each g

E

Z~

has a

with dk

E

_{(0 k}

_H)

and

_(0,0)T

for 0. These number

systems

form the

_object

of this _paper. In

_particular,

we want to

_study

the so-called

_fundamental

domains of these number

_systems.

The

_fundamental

domains of a number

_system

(M,

is defined

_by

Sloppily

spoken, 7

contains all elements of

_{R ,}

with

_integer

_part

zero in

their "M-adic"

_{representation.}

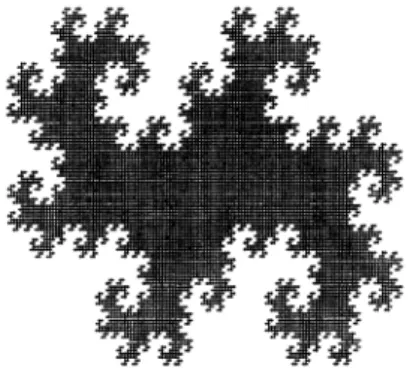
In

_Figure

1 the fundamental domain

corre-sponding

to the M-adic

_{representations}

_arising

from the Gaussian

_integer

-1 + i is

_depicted.

This so-called "twin

_dragon"

was studied

extensively

by

Knuth in his book

_[13].

FIGURE 1. The

_fundamental

domain.

_of

a numbers

_system

Fundamental domains of number

_systems

have been studied in various papers. Kitai and

K6rnyei

[9]

proved,

that T is a

_compact

set that

tesse-lates the

_plane

in the

_following

way.

Furthermore,

we want to

_mention,

that the

_boundary

of ~’ has fractal dimension. Its Hausdorff and box

_counting

dimension has been calculated

by

Gilbert

_[4],

Ito

_[7],

_{MiiHer-Thuswaldner-Tichy}

_[16]

and Thuswaldner

_[17].

In the

_present

_paper we are interested in

topological

properties

of Y. Before we

give

a _survey on our results we shall define some basic

_objects.

Let S

Then

_by

₍₁₎

the

_boundary

of F has the

_{representation}

.,

-Hence,

the

_boundary

of Y is the set of all elements of

_F,

that are contained

in F

_{+ g}

for a

certain g 0

(0,0).

Of _course, 8J’ _may contain

points,

that

belong

to F and two other different translates of 7. These

_points

we call

vertices of F. Thus the set of vertices of F is defined

_by

In Section 2 we

_study

the set of vertices of ~. It turns

_out,

_that,

_apart

from one

_exceptional

_case, J’ has at least 6 vertices. In some cases we derive

that V is an infinite or even uncountable set. In Section 3 we _prove the

connectedness of J’ and show that each element of

_T,

which has a finite

M-adic

_expansion,

is an inner

_point

of T.

2. VERTICES OF THE FUNDAMENTAL DOMAIN T

In this section we

_give

some results on the set of vertices V of F. For

number

_systems

_emerging

from Gaussian

_integers,

similar results have been established with

_help

of different methods in Gilbert

_[3].

We start with the definition of useful abbrevations. Let

be the M-adic

_{representation}

_{of g.}

_Note,

that the

_digits

_dj

_{(-Hl j H2)}

are of the

shape

_{dj =}

E Thus for the

_expansion

₍₃₎

we will

write

If the

_string

cl ... cH

occurs j

times in an M-adic

_{representation,}

then we

write

_{[ci ... cH]j .}

If a

representation

is

ultimately periodic,

i.e. a

_string

cl ... cH occurs

_infinitely

_often,

we write

[Cl

... First we

show,

that

for A &#x3E; 0 _any fundamental domain F contains at least 6 vertices.

Theorem 2.1. Let

_{(M, ~(,IU))}

be a number

_system

which is induced

by

the base b

_of

a canonical number

_system.

Let

Pb(x) =

x 2

+Ax+B

with

A &#x3E; 0 be the minimal

_{Polynomial of}

b. Then the set

_of

vertices V

_of

the

fundamental

domain F

_of

this number

_system

contains the

_points

Depending

on the cases A =

1,

1 A B and A =

B,

the

points

Pi

The case A = 1 is very similar _to the _case 1 A

B;

just replace

the

representation

1(A - 1)(B - A+ 1)

by

11(B - 1)0

in the above table. Remark 2.1.

_Note,

that we have 0 A B &#x3E; 2. Hence the

digits

of the

6

_points

indicated in Theorem 2.1 are all admissible.

Proof of

the theorem. We will _prove that each of the 6

_{points Pl, ... , P6}

is

contained in three different translates of

_7,

as indicated in the statement

of the theorem. First we consider the

point Pl.

Write a? = -~.

_By

_using

b2 +

Ab + B =

0,

_{we see} that

are formal

_{representations}

of zero.

Adding

the second

_{representation}

for 0

given

in

₍₄₎

_twice,

we have

For A B this

_yields

For A = B the last

expansion

lA.((B -1)0(A -1)~~

is not admissible since

A &#x3E; B - 1. In order to settle this case we use the first

representation

of zero

_given

in

(4)

to

_get

1A = 1B = 1B ₊

1(B - 1)OB

=

1(A - 1)10.

As _a

result,

we have

for A = B. Since

P2 = MP1,

_we

get

the desired results also for

P2.

Now

we treat

which

_implies

for A B and

for A = B. Since F

permits

_an involution i

J’ is

_symmetric

with

_respect

to the center

~

this map sends each 1 . Thus we have

for A B and

for A = B.

_Furthermore,

_{it is easy} to see, that

cp(P1)

=

P4,

p(P2 )

= P5

and

P6

Thus also

_{P4, P5}

and

P6

are vertices of F that are contained

in the translates of F indicated in the statement of the theorem. C1

In the case A = 0 it is easy _{to see} that J’ is _{a square.} It has

exactly

4

vertices. These are the "usual" vertices of the _square. Thus we

only

have

to deal with the case A = -1. We will folmulate the

corresponding

result as a

_corollary.

Corollary

2.1. Let the same

_settings

as in Theorem 2.1 be in

force,

but

assume _rtow, that A = - 1. Then the

following

table

_gives

6

points

Pi

(1 j

6),

that are contained in the set

_of

vertices V

_of

~’.

_Furthermore,

we

_give

the translates T + w, to which

Pj

belongs.

Proof.

Let be bases of number

to

_M1

and

_{Mz, respectively.}

We know the vertices from Theorem 2.1

and will construct the vertices of

_.~2

from it. To this matter let

_M1

=

easy to see, that then

M2

=

with

_G2

=

diag(-I, I)Gi.

Now _suppose, that

M1kaj

_E

F1

n

F1

₊

fl

_{(Wl, W2 )T}

with _{V1,V2,W1,W2 E} Z is a vertex of

_{Fl. Using}

the

_fact,

that for _{ak E} .M and

_setting

d =

0.[O(B - 1)]00

we

_easily

derive that

Observe,

that

_by

the selection of

_d,

_Q

has an admissible

M2-adic

rep-resentation with

_integer

_part

zero. Thus

Q

E

_~2.

Since _any

ele-ment of

_T2

can be constructed from elements of

1=1

in the same _way we

conclude,

that

_T2

= ₊ d. But with that

(5)

reads

Q

E +

(-vl, v2)T

n T2

+

(-W1,W2)T.

Thus

_Q

is a vertex of

.~2.

The

_{representations}

in the table

_above,

can now

_easily

be obtained from

the results for A = 1 in Theorem 2.1. D

The

_following

_corollary

is an immediate _consequence of Theorem 2.1 and

Corollary

2.1.

Corollary

2.2. For 1 A B we have

while

_for

A = 1

holds.

Remark 2.2.

_Note,

that "~" may be

replaced by

"=" in

Corollary

2.2 if

2A B + 3. This is shown for the Gaussian case in

[16].

For

_arbitrary

quadratic

number fields this fact can be

proved

in a similar _way.

Theorem 2.2. Let the same

settings

as in Theorem 2.1 be in

force. If

2A = B _-f- 3 then T has

.

Then,

using

i

Here we _{set a? = -x,} as before. We will

_show,

that the

_points

are vertices of F. Therefore we need the

_{representation}

_(6).

With

_help

of this

_{representation}

we define the

_following

_{representations}

of zero.

J ...JJ ,- - , i

In the

_sequel

we write

_kXj

(k

E

_Z)

if we want to

_multiply

each

_digit

of the

_{representation}

_Xj

_by

k.

_Furthermore,

addition and subtraction of

representations

is

_always

meant

_digit-wise.

After these definitions we define

the

_following,

more

complicated

_{representations}

of zero.

, , , _ ..

Finally,

we

_observe,

that

_{for j}

E N

and this

_implies

_Qj

E V. It remains to

_show,

that the elements

_1,

are

_pairwise

different. This follows from the

following

observation. Select

k E N

_arbitrary

and let

_{j1, j2}

k.

_Suppose,

that

_Qj,

and

_Q~2

are

repre-sented

_by

the

_{representation}

₍₇₎

_{for j}

_{= jl}

_{and j}

=

j2,

respectively.

Then

Qj,

= and

_only

if

M6k+2Qj¡

= For k &#x3E;

max(ji, j2),

M6k+2Qj¡

and

_M6k+2Qj2

have the same

digit string

[O(B -

after the comma.

_Hence,

_they

can

_only

be

_equal,

if their

_integer

_parts

are

equal.

But since

_(M,

_4l(N))

is a number

_system,

this can

only

be the _case, if the

digit strings

of their

_integer

_parts

are the same. This

_implies

_j,

=

j2.

So

can be selected

_arbitrary,

the result follows. Thus we found

infinitely

_many

different vertices of J’. D

Theorem 2.3. Let the same

settings

as in Theorem 2.1 be in

force. If

2A &#x3E; B + 3 then Y has

_uncountably

_many vertices.

using (6),

we see that

Thus is a vertex of .F’. Fix an

_integer

k,

such that all

eigenval-ues of

Mk

are

_greater

than 2

(such

an

_integer

_exists,

since the

eigenval-ues of M are all

_greater

than

1).

This

implies,

that the

representations

cj E

_~0,1}

_{(j &#x3E;}

₁₎

_represent

_pairwise

different

el-ements

ofIae2

for different

_{{O, 1}}

_sequences

_{Because g + K}

_B-1,

each of the

_uncountably

_many

_{representations}

corresponds

to a vertex Since

_they

are

_pairwise

_different,

the theorem

is

_proved.

0

3. CONNECTEDNESS AND INNER POINTS OF THE FUNDAMENTAL DOMAIN 0

In this section we will

show,

that the fundamental domain F is arcwise

connected. To establish this

_result,

we will

apply

a

_general

theorem due

to Hata

_(cf.

_{[5, 6])}

which assures arcwise connectedness for a

large

class

of sets. The second result of this section is devoted to the structure of the inner

_points

of ~’. In

_particular,

we _prove, that each

_point

with finite

M-adic

_{representation}

is an inner

_point

of Y. In this section we will use

the notation

We start with the connectedness result.

Theorem 3.1. Let

_(M,

_4l(N))

be a nurnber

_systems

which is induced

by

the base b

_of

a canonical numbers

_system

in a

quadratic

numbers

field.

Then the

_fundamental

domain 7

_of

_(M,

is arcwise connected.

Furthermore,

Theorem 2.1

_implies

that .

sets contained in the union of

₍₈₎

form a chain in the sense that

(T

+

g)

fl

_(:F

+

_(g

+

_(1,

O)T)) 0

0

for _g E

_{4l(N) )}

_{(B -}

1, 0)T .

Thus T fulfills the conditions

_being

_necessary for the

_application

of a theorem of

Hata,

namely

[5,

Theorem

_4.6~.

This theorem

_yields

the arcwise connectedness of T. D Now we _prove the result on the inner

_points

of T.

_Note,

that the existence

of inner

_points

is an immediate _consequence of

[9,

Theorem

1].

Theorem 3.2. Let

_(M,

_(k(AO)

be a number

_systems

in

}R2,

which is induced

by

the base b

_of

a canonical numbers

_system

in a

_quadratic

number

field.

Then

_for

each k E N we have

Proof.

First we will

_show,

that 0 is an inner

_point

of T.

_Suppose,

that 0 is

contained in the

_boundary

of T. Then

_by

₍₂₎

there exists a

representation

of zero of the

_shape

This

_{representation}

_implies

0 + If we

multi-ply

(9)

by

Mj

_{for j}

E N

_arbitrary,

we

conclude,

that 0 E :F +

CHiCHI-1 ... ClCOC-1 ...

C_j for

each j

E N.

Hence,

0 is contained in

in-finitely

many different translates of ,~’. But since T is a

_compact

set this

is a contradiction to

_(1).

Thus 0 E

_int(.F).

Now fix k E N‘

_{and g}

E

_Tk.

Then 0 E

_{int (.F)}

_{implies, that g}

E

int(M-k.F

+

_g).

The result now follows from the

representation

There is a direct alternative

proof

of this theorem

by

using

the methods

of

_[1]

and

_[2].

In these _papers a similar result for the

tiling

generated

by

Pisot number

_systems

is shown.

REFERENCES

[1] S. _{Akiyama, Self affine tiling} and _pisot numeration system. Number Theory and its

Appli-cations _{(K. Györy} and S. _{Kanemitsu, eds.),} Kluwer Academic Publishers, 1999, pp 7-17.

[2] S. _Akiyama and T. Sadahiro, A self-similar tiling generated by the minimal _pisot number. Acta Math. Info. Univ. Ostraviensis 6 _(1998), 9-26.

[3] W. J. _{Gilbert, Complex} numbers with three radix representations. Can. J. Math. 34 (1982),

1335-1348.

[4] Complex bases and fractal similarity. Ann. sc. math. _Quebec 11 _(1987), no. _1,

65-77.

[6] Topological aspects of self-similar sets and singular functions. Fractal Geometry

and Analysis (Netherlands) (J. Bélair and S. Dubuc, eds.), Kluwer Academic Publishers,

1991, pp. 255-276.

[7] S. Ito, On the fractal curves induced from the _complex radix _expansion. Tokyo J. Math. 12

(1989), no. _2, 299-320.

[8] I. Kátai, Number systems and fractal geometry. preprint.

[9] I. Kátai and I. Környei, On number systems in _algebraic number _fields. Publ. Math. Debre-cen 41 _(1992), no. _3-4, 289-294.

[10] I. Kátai and B. Kovács, Kanonische _{Zahlensysteme} in der Theorie der Quadratischen

Zahlen. Acta Sci. Math. _(Szeged) 42 _(1980), 99-107.

[11] _ Canonical number systems in imaginary quadratic fields. Acta Math. Hungar. 37

(1981), 159-164.

[12] I. Kátai and J. Szabó, Canonical number systems for complex integers. Acta Sci. Math. (Szeged) 37 _(1975), 255-260.

[13] D. E. Knuth, The art of computer programming, vol 2: Seminumerical algorithms, 3rd ed. Addison Wesley, London, 1998.

[14] B. Kovács, Canonical number _{systems in algebraic} number _fields. Acta Math. _Hungar. 37

(1981), 405-407.

[15] B. Kovács and A. _Pethö, Number _systems in integral domains, especially in orders _of alge-braic number _fields. Acta Sci. Math. _(Szeged) 55 _(1991), 286-299.

[16] W. _Müller, J. M. Thuswaldner, and R. F. Tichy, Fractal properties of number systems.

Peri0dica Mathematica Hungarica, to appear.

[17] J. M. Thuswaldner, Fractal dimension _of sets induced by bases of imaginary quadratic fields,

Math. Slovaca 48 _(1998), no. _4, 365-371.

Shigeki AKIYAMA

Department of Mathematics

Faculty of Science

Niigata University

NIIGATA - JAPAN

E-maal : _{akiyamaQmathalg . ge . niigata-u. ac . jp}

J6rg M. THUSWALDNER

Department of Mathematics and Statistics Montanuniversitat Leoben

Franz-Josef Str. 18 LEOBEN - AUSTRIA