Bridging conduction and radiation : investigating thermal transport in nanoscale gaps

ARGIUVES

OF TECHNOLOLGY

APR 15 2015

LIBRARIES

Bridging Conduction and Radiation: Investigating Thermal

Transport in Nanoscale Gaps

by

Vazrik Chiloyan

Submitted to the Department of Mechanical Engineering

in partial fulfillment of the requirements for the degree of

Master of Science in Mechanical Engineering

at the

MASSACHUSETTS INSTITUTE OF TECHNOLOGY

FEBRUARY 2015

Massachusetts Institute of Technology 2015. All rights reserved.

AuthorSignature

redacted

A uthor

...

... ...

Department of Mechanical Engineering

January 16, 2015

Certified by ...

Signature redacted

Gang Chen

Carl Richard Soderberg Professor of Power Engineering

Thesis Supervisor

Signature redacted

Accepted by ...

...

David E. Hardt

Chairman, Department Committee on Graduate Students

Bridging Conduction and Radiation: Investigating Thermal

Transport in Nanoscale Gaps

by

Vazrik Chiloyan

Submitted to the Department of Mechanical Engineering on January 16, 2015, in partial fulfillment of

the requirements for the degree of Master of Science in Mechanical Engineering

Abstract

Near field radiation transfer between objects separated by small gaps is a widely studied field in heat transfer and has become more important than ever. Many technologies such as heat assisted magnetic recording, aerogels, and composite materials with interfacial transport involve heat transfer between surfaces with separations in the nanometer length scales. At separations of only a few nanometers, the distinction between classical thermal conduction and thermal radiation become blurred. Contact thermal conduction is understood through the means of interfacial transport of phonons, whereas thermal radiation is understood by the exchange of heat through the electromagnetic field. Typically conductance values in the far field radiation regime are on the order of 5 W/m2

K, whereas contact conductance is on the order of 108 W/m2K. While near field radiation experiments have reached separations down to on the order of 10 nm and measured 104 W/m2

K, there are still 4 orders of magnitude change that occurs over 10 nm of separation. However to this day, there does not exist a single unified formalism that is able to capture the relevant physics at finite gaps all the way down to the contact limit.

The success of the continuum electromagnetic theory with a local dielectric constant has allowed accurate modeling of thermal transport for materials separated by tens of nanometers. The validity of this approach breaks down at the contact limit as the theory predicts diverging thermal conductance. The nonlocal dielectric constant formalism has successfully been applied to correct this error and predict transport at nanometer separations for metals and nanoparticles. However, success has been limited for deriving nonlocal dielectric constants for insulators as it is both theoretically and computationally more challenging and requires accurate atomic modeling to retrieve a valid continuum dielectric that reproduces the response of the system. In this work, the continuum approach is avoided and an approach is taken which more closely resembles the conduction picture, by performing atomistic modeling of the thermal transport between two semi-infinite media. The interatomic forces of both short-range chemical bonding forces and long ranged electromagnetic forces are included in an atomistic Green's function formalism in order to accurately calculate thermal transport at finite gaps down to the contact limit. With a single, unified formalism the bridge between conduction and radiation is finally achieved.

Thesis Supervisor: Gang Chen

Title: Head of the Department of Mechanical Engineering and Carl Richard Soderberg Professor of Power Engineering

Dedication

Acknowledgements

I would like to start off by thanking my advisor, Professor Gang Chen, for all that he has done in

the past several years in helping shape me as a good scientist and a capable researcher. His ambition and drive are truly inspirational, and his fearless approach to tackling hard problems is one of the most valuable lessons I have learned from him. I would also like to thank Professor Sheng Shen, with whom I had the pleasure of doing research with in the NanoEngineering group as an undergraduate student at MIT while he was a graduate student in the group. It was during those initial years that I "got my hands dirty" and jumped into research, as Professor Chen always says, that taught me to be patient and to be perseverant.

I would like to thank Dr. Keivan Esfarjani and Dr. Jivtesh Garg for all of their time and

support throughout the past years working together on this project. I have spent countless hours discussing and learning from them, and am always thankful for their insight and patience. My first year as a graduate student I had the pleasure of working with them directly, and it was one of my fondest years yet in graduate school. I would not be where I am now if it weren't for these fantastic mentors. I would also like to thank Mr. Bolin Liao and Mr. Sam Huberman for the general discussions we have shared bouncing ideas around, and for their help in improving my work and writing for this thesis. I would also like to thank Dr. Kenneth McEnaney and Dr. Kimberlee Collins for their care and wisdom; I have always been able to turn to them for help. As senior graduate students, they are truly members of the group that I try to model myself after. Last but not least, I would like to thank the members of the NanoEngineering group in general for all of the insightful discussions and happy moments shared together. I can gladly say I have

learned something and shared a good laugh with each and every one of you, and hope I have done the same for you.

Finally I would like to thank my beloved family and friends for all of their support. They have helped me keep perspective in graduate school, and have pushed me past any low points that I have encountered. Their unwavering confidence in me constantly impresses me, and

Contents

1 Introduction 13

1.1 Fluctuating electrodynamics and macroscopic Maxwell equations ... 14

1.2 Atomic formalism and microscopic Maxwell equations ... 26

1.3 Organization of thesis ... 30

2 Force Constants 31 2.1 Short-range force constants... 32

2.1.1 Spring constants and phonon dispersion ... 32

2.1.2 Sodium chloride short-range force constants ... 36

2.2 Electrom agnetic force constants ... 39

2.2.1 Fields of single m oving charge ... 41

2.2.2 Summ ation of forces for crystal slab ... 47

3 Lattice Dynamics and Green's Functions 54 3.1 Classical transport through atom ic chain... 57

3.2 Quantum transport through crystal slabs ... 64

4 Heat transfer between Sodium Chloride slabs 80 4.1 Num erical interpolation ... 80

4.1.1 Frequency m esh (I D) ... 81

4.1.2 W avevector m esh (2D) ... 85

4.1.2.1 Irregular total Brillouin zone ... 85

4.1.2.2 Irregular irreducible Brillouin zone ... 88

4.2 Convergence analysis... 93

4.2.1 Slab size ... 93

4.2.2 W avevector m esh... 97

4.2.3 Frequency m esh ... 100

4.3 Num erical Results...102

4.3.1 Continuum form alism results...102

4.3.2 Atom ic formalism results and com parison ... 113

5 Conclusion 122 5.1 Sum m ary... 5.2 Future work... References...125

List of Figures

1-1 Schematic of a wave hitting a surface at an oblique angle...15

1-2 Schematic of thermal radiation transfer between two objects. The far field (a) for which the transfer is by propagating waves, for which evanescent fields (b) do not contribute, and the near field (c) for which evanescent fields couple and allow for tunneling...16

1-3 Schematic of thermal radiation between two objects exhibiting surface waves. The two sided evanescent fields decay on either side of the interface, and for large separations (a) do not couple the slabs but for small enough separations (b) the fields decaying from one material can penetrate into the second medium to transfer energy. ... 17

1-4 Heat transfer between glass substrate and glass microspheres...21

1-5 Heat transfer for various materials demonstrating the enhancement of thermal transport with the presence of phonon-polaritons. ... 23

2-1 Schematic of monoatomic linear chain with nearest-neighbor coupling. ... 34

2-2 Monoatomic chain dispersion with non-monotonic behavior due to next nearest neighbor force effects ... 35

2-3 Dispersion of a diatom ic chain ... 36

3-1 Schematic of a ID chain coupled to hot and cold thermal reservoirs...57

3-2 Schematic of the sodium chloride structure depicting the left and right heat baths as well as the device region, comprised of the left and right slabs separated by a finite gap...66

4-1 Schem atic of linear interpolation... 83

4-2 Schematic of quadratic interpolation... 84

4-3 Schematic of the irregular total Brillouin zone discrete mesh.. ... 89

4-4 Sodium chloride structure as a rock-salt crystal...90

4-5 Various reflection symmetries of the square lattice crystal...91

4-6 Schematic of the irregular, irreducible Brillouin zone...92

4-7 Convergence with respect to number of layers in transmission...95

4-8 Convergence with respect to the number of layers in conductance. ... 96

4-9 Convergence with respect to number of layers for larger wavevector mesh. ... 97

4-11 Convergence study with respect to wavevector studied the (a) transmission function and

(b) integrated conductance at contact limit (0 gap)...99

4-12 Convergence study with respect to wavevector studied the (a) transmission function and

(b) integrated conductance at 2 layer gap (0.56 nm)...99

4-13 Convergence study with respect to wavevector studied the (a) transmission function and

(b) integrated conductance at 5 layer gap (1.40 nm)...100

4-14 Convergence study with respect to frequency mesh. ... 101 4-15 A simple schematic of a damped harmonic oscillator...104

4-16 Schematic of a crystal lattice with the spherical region separating atoms that contribute to the macroscopic field vs. the local microscopic contribution. ... 106

4-17 Computed real part of dielectric constant and analytic fitting to the Drude-Lorentz m odel...Il 4-18 Computed conductance in continuum Rytov formalism with analytic fitting for the

representative temperatures of T = 110 K and T = 55 K...113

4-19 Comparison of atomic calculation to continuum Rytov calculation. Plot in log-log form to show convergence to power law decay pattern for larger gaps...114 4-20 Comparison of atomic calculation to continuum Rytov calculation. Plot in semi-log

form to show divergence of Rytov formalism at contact limit with finite conductance in the atomic formalism at contact limit of bulk sodium chloride structure...115 4-21 Transmission function integrated over the wavevector mesh for the gaps of (a) contact,

(b) 0.28nm, (c) 0.56nm, (d) 1.13nm, (e) 1.69nm, (f) 2.81 nm. ... 116 4-22 Bulk phonon dispersion for sodium chloride. ... 117

4-23 Transmission function integrated over the frequency mesh for the gaps of (g) contact,

Chapter 1

Introduction

Planck predicted that the results valid at the far field for thermal radiation break down when the linear lengths and radii of curvature of bodies are comparable to the photon wavelength [1]. With the improvement of experimental techniques and equipment, the breakdown of Planck's blackbody radiation law has been demonstrated [2]. A theoretical framework for studying near field radiation transport for systems separated by distances of several hundred nanometer and larger was developed by Rytov [3,4] and applied first to the problem of thermal transport between two semi-infinite media by Polder and van Hove [5]. The fluctuating electrodynamics approach, which utilizes the continuum electromagnetic theory with a local dielectric constant, has improved understanding of thermal transport down to several nanometer spacings. However the push to even smaller spacings continues!

The need to understand thermal transport at separations of a few nanometers and smaller is as important as ever. Technology is shrinking in size, and now resides in the several nanometer length scales where the current theory is not sufficient for describing the relevant physics for the thermal transport. Heat assisted magnetic recording devices now have separations between substrate and writing head of only a single nanometer [6]. Aerogels have been utilized in thermal considerations due to their ability to provide a cheap, lightweight, and low thermal conductivity material. Aerogel porosity is such that particles have separations on the order of a

few nanometers, and are relevant for the transport regime described [7]. Furthermore,

composite materials formed by multiple components with interfaces can have interface separations that can range from contact to several microns [8]. Being able to describe the

thermal transport across the total range of gaps with a single formalism can lead to greater insight at the dependence of transport on the gap and a better understanding with which to design interfaces to control transport. While the atomistic modeling of thermal interface transport has been done considering only short-range forces [9], the modeling of longer ranged electromagnetic forces that exist at these larger length scales is not complete.

In this chapter we aim to give a brief introduction to research in near field radiation and outline the modem method of analysis using fluctuating electrodynamics using continuum modeling with a local dielectric constant. This will allow us to formally introduce the problems that arise and the approaches taken to alleviate the issues. Henceforth, we will introduce the atomic formalism utilized to calculate thermal conduction, and explain how the formalism will be extended to handle near field radiation to improve understanding of thermal transport at nanoscale gaps.

1.1 Fluctuating electrodynamics and macroscopic Maxwell equations

We begin with a brief overview of far field and near field radiation transfer. Consider two semi-infinite bodies separated by a finite gap. When an electromagnetic wave is excited within one material, when it reaches the interface it can be both transmitted and reflected. The boundary condition describing the angle of the transmitted beam relative to the incident beam is given by Snell's law [10]:

where n,2 are the refractive indices of the materials, given by n12 = E12 / -- where EI2 are the

dielectric constants of the material.

B1

Figure 1-1: Schematic of a wave hitting a surface at an oblique angle. Figure adapted from

Ref. [II].

When the incident angle is smaller than the critical angle given by 0, = arcsin (n2 / n), there

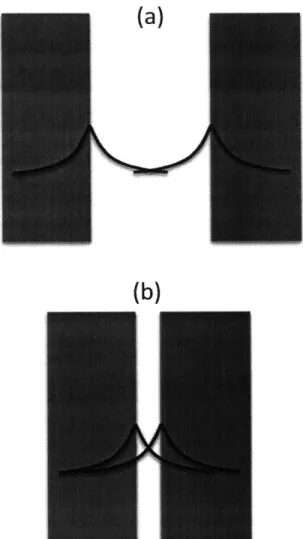
will be a wave transmitted as well as reflected by the interface. However, when the incident angle is larger than the critical angle, total internal reflection occurs, so that there is no transmitted propagating wave and instead the electromagnetic field in the second medium is evanescent and decays exponentially from the interface. The far field regime is the regime for which the spacing between the two bodies is much larger than the wavelength of the electromagnetic radiation, so that the evanescent waves do not contribute at these separations. Figure 1-2(a) shows the transfer across the gap by propagating waves that travel unhindered in space and transmit across the interface. At these large gaps, the evanescent fields decay and cannot contribute to thermal transfer to the second material as shown in Fig. 1-2(b). When the

spacing between the materials decreases, the evanescent field can couple across the gap to the

second material and allow for tunneling of the wave in the near field regime (see Fig. 1-2(c)).

a

TOSON

*0

Figure 1-2: Schematic of thermal radiation transfer between two objects. The far field (a) for

which the transfer is by propagating waves, for which evanescent fields (b) do not contribute,

and the near field (c) for which evanescent fields couple and allow for tunneling. Figure adapted

from Ref. [12].

For the cases shown so far, the electromagnetic field was propagating on one side of the

interface and evanescent on the other. It is also possible to have surfaces waves, for which the

electromagnetic field travels only along the surface and exhibits exponential decay on both sides

of the interface, thus enhancing the energy density near the interface as no energy propagates

into the material. Figure 1-3 shows that in the far field regime, the fields decay in the vacuum

between the materials and do not contribute to heat transfer. However, for small enough

spacings, the fields from one material penetrates into the second material and yields coupling

between the slabs so that it allows for the transfer of heat between the two systems.

(a)

(b)

Figure 1-3: Schematic of thermal radiation between two objects exhibiting surface waves. The

two sided evanescent fields decay on either side of the interface, and for large separations (a) do

not couple the slabs but for small enough separations (b) the fields decaying from one material

can penetrate into the second medium to transfer energy.

To explain the possibility of having one-sided and two-sided evanescent waves, we observe the behavior of the wavevector component in the cross plane direction, k, (see Fig. 1-1 for

coordinate system). The dispersion of light in a given medium is linear, given by w=v , where v = c / n so that the speed of light is smaller in a medium than in vacuum. The wave

vector of the electromagnetic field can be described ask = ,+ k, where k, describes the wavevector in plane to the semi-infinite medium and k- describes the wavevector normal to the interface (see Fig. 1-1). The dispersion relation requires that the wavevector in the z-direction

satisfy:

2

2

k,= C2 -k Pk, _P (1.2)

where we have utilized the relation between the dielectric constant and index of refraction for a non-magnetic material n = VE/ E0 . When k, is real, the field is propagating into the given

medium, and when it is imaginary, the wave eike-i' = e e icze'''' will describe exponential decay in the z-direction and propagation can occur only along the surface in the second medium. When the dielectric constant E> 0, the case of total internal reflection with a one-sided

evanescent wave can occur when the angle is larger than the critical angle so that k, is real in the medium of dielectric constant r > 0 whereas it is imaginary in the vacuum E = 0 due to the

continuity of k, across the interface as required by the boundary conditions of the electromagnetic fields [10,11]. The case of surface waves yield two-sided evanescent modes, since the dielectric constant of such modes is such that c <0 and k, is large [13-15]. Within the

medium, E <0 makes k, imaginary, whereas in vacuum, the large k, of the surface waves

makes k, imaginary yielding two sided evanescent waves.

There has been a great push both theoretically and experimentally to understand the near field regime and the contribution of heat transfer from these evanescent fields. The understanding of thermal transport through radiation in the near field regime was greatly enhanced with studies beginning in the 1960s. There were theoretical and experimental investigations of near field radiation between metals with the goal of understanding the enhancement of radiation at close spacings for metals with the pioneering work of Boehm, Cravalho, Domoto, Tien et. al [16-19]. Hargreaves measured and predicted anomalous transport with the decrease of spacing between metal plates on the order of micron gaps [20].

In the 1950s and 1960s, with the work of Rytov, the link between Maxwell's equations and thermal transport in the quantum regime was provided by the fluctuation-dissipation theorem, relating the fluctuations of the electromagnetic field to the temperature [3,4]. The formulation developed by Rytov, often known as fluctuating electrodynamics, views the problem of thermal radiation transport between two mediums with a continuum model. The two interacting media are modeled using macroscopic continuum descriptions such as a frequency dependent dielectric constant e((o) , characterizing the response of the bulk system to an externally imposed electromagnetic field. The electromagnetic fields are generated due to the vibration of molecules within the systems from thermal fluctuations. Therefore, the finite temperatures induce interactions between the slabs and result in a net transport of heat through electromagnetic waves. Polder and van Hove utilized the fluctuation-dissipation theorem the first time calculating the transport between two semi-infinite materials [5]. Since its development, the formulation has been extensively utilized to understand transport between nanoparticles and

closely spaced slabs to accurately predict the enhancement in heat transfer in the near field

regime [21-24].

On the experimental side, there was a great push to reaching smaller and smaller gap spacing measurements in order to understand what happens to the behavior of thermal radiation over a wide range of spacings all the way down to contact. Planar measurements of surfaces down to single micron separations were performed of between non-metallic surfaces in the case of quartz [25] and also for sapphire [26]. Due to the difficulty of alignment of planar surfaces at gaps below micrometer range, Narayanaswamy et. al. utilized a sphere-plate geometry to be able to observe distances down to 30 nanometer separation [27,28]. The utilization of bi-material cantilevers allowed for spacings on the order of tens of nanometers due to the high thermal sensitivity of bi-material cantilevers [12-14,29].

Figure 1-4 shows calculated and measured radiative heat transfer between two parallel plates, with the experimental data converted from a sphere plate experiment. Planck's blackbody radiation law predicts that thermal transport between two ideal semi-infinite blackbodies separated by vacuum is proportional to

(T-i-T4,

between the bodies as it accounts only for the propagating modes. This is due to the rapid exponential decay of the evanescent modes which do not contribute until the spacing between the materials has reached on the order of microns or smaller [2,14,30-33]. As the separation becomes smaller, the heat transfer increases rapidly due to contributions from the evanescent waves.

2500 --. 4

10. .

01

E 01 ,0 2

200oo parallel plates 0

a' 1500 -

-810.01

8

4

Figure 1-4: Heat transfer between glass substrate and glass microspheres of diameter 100 microns (blue circles) and 50 microns (violet triangles). Figure adapted from Ref. [28].

Figure 1-4 shows that the far field limit of (T4 - T2) is achieved by a separation on the order of

10 microns, after which point there is no dependence on distance. However for smaller gaps, the

transport exceeds the blackbody limit by several orders of magnitude due to the contribution of the evanescent modes. Greffet et. al. highlighted one mechanism for the large enhancement of thermal radiation at small length scales observed due to resonant surface waves coupling known as surface phonon polaritons between the closely spaced materials to achieve 104 for the thermal conductance [23,24,30,34]. For non-metallic surfaces that can support these resonantly coupled modes, the heat transfer is enhanced by an even larger factor than simply that due to evanescent waves coupling the surfaces [28]. For materials that do not support these resonantly coupled modes, the heat transfer saturates at n2 times the blackbody limit due to the contribution of the far field as well as the non-resonant evanescent modes that tunnel from the total internally

reflected light shown in Fig. 1-2 [13,14,35]. In this case, the fields are evanescent only on one side of the interface and propagating on the other side (see Fig. 1-2). For surface polariton modes, the fields are evanescent on both sides of the interface (see Fig. 1-3) due to the resonant condition within the material (e = -te) satisfying a negative dielectric constant [13,28,36]. The two sided exponential decay from the interface has been shown to result in a large energy density located at the surface [15,28,36] and leads to the large enhancement of thermal transfer in the near field regime beyond that from non resonant evanescent waves. The enhanced density of states localized at the surface is shown in Fig. 1-5 arising from the surface phonon polariton waves.

xlO 2.5 .. 2 S102 Y --- si Au -E 1.5 - AU U) o 1 d=50nm-0.5 0-10 _{Wavelength (jim) 2} i02 -x10 . B - -- '02 - S E2- _{.... Ss0} 2 - Au 4-2 d d=50nm 10 Wavelength (m) 102 1 C --'02 E - - - S02 -S

~10

Figure 1-5: Heat transfer for various materials demonstrating the enhancement of thermal transport with the presence of phonon-polaritons. The existence of resonances (a) in the modes that transport yield large enhancements (b) in the thermal transport for those given wavelengths, and result in enhancements by orders of magnitude (c) over materials that do not support such resonant couplings. Figure adapted from Ref. [28].

Figure 1-5 shows the enhancement of thermal transport in the near field for various materials that can support surface phonon polariton and ones that cannot. The figure also demonstrates the importance of both materials being able to support the wave, so that materials with mismatched resonances will not have a large enhancement.

For the case of metals, the analogous waves are surface plasmons as the resonant surfaces waves due to electrons coupling closely spaced metal surfaces [31,37,38]. Phonon-polaritons

couple the surfaces together due to the resonant interaction between photons (light) and phonons in the material (vibrations of the solid) [2,13,14,27,28,30]. The ability for the materials to support these resonant surface waves results in a large enhancement in thermal transport between polar materials which doesn't saturate as demonstrated by Fig. 1-4 [39].

The theoretical formulation developed by Rytov accurately captures heat transport down to nanometer length scales and has successfully predicted thermal transfer in the near field regime [27,28,30,31]. However, at small enough separations, the gap spacing becomes comparable to the atomic spacings within the materials. In this case, the approximation of treating the slabs as continuum becomes invalid. Furthermore, the electromagnetic coupling and enhancement discussed within the Rytov formulation only captures the effect of long ranged electromagnetic forces. At contact, the mode of heat transfer is by thermal conduction and is dominated by short-range interactions which generate the vibration spectrum of the crystal [40,41] and are much greater in magnitude than the electromagnetic long ranged forces. The contribution of phonons to thermal transfer will be expanded upon in the next section.

The local dielectric approach, which assumes only frequency dependence, E(co), and no dependence on the wavevector for the system's response to electromagnetic waves, fails to capture transport at sub-nanometer scales. This is exhibited by the divergence predicted going to the contact limit. The local dielectric constant approach yields power law behavior proportional to d-2 _{. A formalism utilizing a non-local dielectric constant has been successfully applied for}

metals and small particles. In this case, the dielectric constant depends on both frequency as well as the wavevector, dropping the assumption that the fields are local in space. This approach has provided improved understanding of the Casimir force between metal plates [42]. The optical properties of materials has been modeled using the non-local dielectric approach [43-47]. The

zero coherence length of the local dielectric constant approach has also been considered with a finite width to alleviate the divergence issue [48] for polar materials. The success has been limited to metals predominantly due to the availability of non-local dielectric constant

models [22].

Given the limitations of utilizing a continuum model at nanometer scale gaps, atomistic modeling was utilized to capture the details of transport at this length scale. Volz et. al. predicted a transition regime for thermal transport at the nanometer regime [49,50]. Utilizing both molecular dynamics simulations as well as the atomistic Green's function formalism, the thermal transport between silica nanoparticles was calculated. The systems were limited only to nanoparticles, and neglected the effects of time retardation of the electromagnetic fields due to small gap studies of small nanoparticles, but predicted a transition regime at small gaps with a faster divergence for sub-nanometer gaps than the traditional d2 . While the transport between

nanoparticles has given some insight into what vibrational frequencies transport across the gap depending on the spacing [50], the transition from radiation to conduction requires extended materials for which the language of lattice waves, phonons, is appropriate.

Molecular dynamics has been utilized as a popular technique within an atomic formalism because it has the advantages of being able to account for interactions between atoms [8]. So long as the appropriate inter-atomic potentials can be obtained, the method can yield thermal transport within the atomic picture. However the method is limited due to the requirement of large simulation sizes to capture extended materials and remove artificial dependence of the simulation size on the thermal transport. The Green's function formalism, while limited only to linear forces between interactions, has the advantage of being able to yield frequency dependent transmission [15,51-53], which leads to greater insight to what vibrational modes are able to

transmit across the gap depending on the spacing between the materials. Furthermore, molecular dynamics simulations are classical, and cannot account for quantum mechanical effects whereas the Green's function atomic formalism can.

To better understand the transition between the classical pictures of thermal radiation and contact thermal conduction, this work develops a unified approach using atomistic modeling with the Green's function formalism that captures both the short-range chemical bonding forces between atoms as well as the long ranged electromagnetic forces by solving the microscopic Maxwell Equations. The formalism is able to capture thermal transport between two semi-infinite crystals valid for the contact limit as well as any gap separating the slabs. Looking at the spectrum of frequencies that contribute to the thermal transport, the formalism is able to demonstrate and explain the transition from conduction to radiation for the original problem of semi-infinite media [5]. In the next section, we lay out the details of the atomic formalism and the classical problem of interfacial resistance in the language of phonons, and describe how the methodology is extended utilizing the Microscopic Maxwell equations to capture transport at finite gaps between the two materials.

1.2 Atomic formalism and microscopic Maxwell equations

Having looked at the problem from the radiation side, where the drive is to go from large spacings down to the contact limit in order to understand the transition that occurs, one can also look at thermal transport at contact between two materials to understand how the formalism can be extended to go beyond the contact limit and calculate transport with a finite gap. While

transport between metals can include both lattice waves and electron transport, transport between two insulators is dominated by the lattice vibrations, i.e. phonons [15,54]. Each material has a vibrational spectrum, called the phonon dispersion, which describe the allowed vibrational states that exist within the structure [40]. At the interface between two materials, lattice waves generated in one medium is scattered by the interface, so that there is some energy that is reflected by the interface, and some energy that transmits through the interface [41,54]. The scattering occurs due to the acoustic impedance mismatch of the two materials, defects at the interface, and roughness at the interface as typically the two mediums do not bond in a perfect planar geometry. This scattering at the interface yields a finite thermal resistance, or equivalently, conductance G, such that the heat flux across the interface is the product of conductance and the temperature difference, in the small temperature difference limit.

Two popular models that have been utilized in calculating interfacial resistance are the acoustic mismatch model (AMM) and the diffuse mismatch model (DMM) [55,56]. The AMM has was originally utilized to understand interfacial resistance between liquid helium and a solid, and was called Kapitza resistance [15,57,58]. It has been extended to calculating transport across solid-solid interfaces as well [37,59,60]. The AMM assumes a perfect planar interface between two materials and assumes a continuum model for the acoustic vibrations within the two media. The material properties are taken to be the bulk properties of each individual material and scattering at the interface occurs due to the mismatch of the acoustic impedances of the two materials, yielding reflection and refraction of the wave analogous to electromagnetic waves described in the previous section. The DMM takes on the opposite assumption where the interface is taken to be perfectly scattering, such that the waves are randomized and emit from the interface at all angles. The DMM has been applied for understanding transport in super

lattices where the system is comprised of two repeating materials in for example an ABAB format to account for scattering at the interface between the two materials [61]. The inaccuracy of these models stems from the assumption of the continuum model and assuming bulk properties for the waves within each media, whereas a more accurate model would consider an atomic picture to account for the details of the material properties of having two semi-infinite

systems joined together with an interface.

While molecular dynamics has been utilized for simulating wave packets generated in one medium to calculate thermal conductance across an interface and has the virtue of capturing complex interfaces where mixing has occurred or the bonding strength of the materials varies, it does not yield spectral information which is critical for understanding which vibrational modes are transferred and what the transport mechanism is. The Green's function formalism is able to provide a spectral transmission function which allows for greater insight in understanding thermal transport across interfaces, even when the interface is not planar and smooth and has complexities such as roughness [9,62].

The atomic Green's function formalism has been used to understand thermal transport through thermal conduction in the form of lattice waves, i.e. phonons [53,62,63]. Fundamentally, the method seeks to calculate the transport from a hot reservoir to a cold reservoir through the intermediate "device" region [62,64]. The device region is taken to include the interface between the two materials, and the hot and cold reservoirs are represented as semi-infinite leads, which numerically are accounted for recursively [65,66]. The atomic formalism takes in as input the force constants of interaction between the atoms, and outputs a spectral transmission function [53], from which the phonon modes which contribute greatest to thermal transport or are scattered most by the interface can be understood [67].

The forces of interaction between atoms typically include the short-range chemical bonding forces, which are truncated artificially to only include a few nearest neighbor shells at most for computational ease. The approach neglects anharmonicity, therefore it is valid only at low temperatures where the harmonic expansion is valid [64]. At large temperatures, the vibrations of the atoms are larger so that the linearization of the force becomes invalid and requires the inclusion of the nonlinear motion of the atoms. Typically in this case one either turns to molecular dynamics simulations (MD) in order to capture the full dynamics [68-71]. Another method is to treat the nonlinearities as small, and to calculate transport in the Green's function formalism perturbatively [52].

The success of the Green's function formalism hinges upon the accuracy of the force constants that correctly capture the physics of the interactions within the material. In this study, the transport across finite gaps is accurately captured if the long ranged electromagnetic forces are implemented in the formalism alongside the usual short-range forces. Often the short-range forces amongst the atoms are derived through the use of empirical potentials that have been developed using data on various materials, or through more sophisticated computational techniques such as density functional theory [72-75]. For the long ranged forces, turning to the atomic picture, this requires calculating the exact electromagnetic field generated by an oscillating charge, and using the fields summed up over the atoms of the systems to be able to capture the net interaction. There is no longer a macroscopic description of the system; the total fields are obtained through the concept of superposition and summing over all of the atoms present in the crystals. The physical picture of polarization is still present; as a charge vibrates due to thermally induced fluctuations, it emits an electromagnetic field, which puts a force on the other charges and causes them to vibrate. The vibrations from the other atoms induce their own

emitted electromagnetic field, which then affects the original atom. Therefore, the atomic picture captures all of the collective phenomena we expect to see from this collective behavior for communication across a gap but has the required detail that is valid down to the contact limit.

1.3 Organization of Thesis

The organization of this thesis is as follows: Chapter 2 includes the detailed description of the force constants used to characterize the interaction between the atoms of the two systems. The language of phonons is introduced to provide the link between the force constants and the vibrational modes of the system. Chapter 3 gives an outline of the atomic Green's function formalism for a simple system for illustration and its implementation for the system studied. Chapter 4 provides the numerical results of this study, which focuses on thermal transport between sodium chloride semi-infinite crystals. The sodium chloride materials support surface phonon polaritons, and by utilizing the same material for both slabs their resonant frequencies are aligned for enhancement of conductance in the near field [28]. Furthermore, the sodium chloride system was chosen since the force constants were available in literature [76]. Chapter 5

Chapter 2

Force Constants

In this chapter, the two types of force constants are formulated for use in the atomic formalism.

The atomic formalism takes in as input the force constants between atoms and outputs the transmission of energy from the hot reservoir to the cold. The accuracy of the atomic formalism and its ability to capture the physics of the near field regime hinges on the accuracy of the force constants inputted. For the transport across a nanometer scale gap, both the short-range chemical bonding forces, which yield the vibrational spectrum of the solid, and the long ranged electromagnetic forces, which provide the connecting link between atoms across the finite gap, are calculated. Including both is critical in capturing the relevant physics needed to accurately describe thermal transport across a gap at nanometer and sub-nanometer spacings.

The chapter will begin with first a description of the link between short-range harmonic force constants and the vibrational spectrum of a crystal. These bonding forces give rise to lattice waves within a solid called phonons. Interfacial transport is calculated within the atomic Green's function formalism by utilizing an accurate input of these short-range bonding forces [9,53]. The inclusion of short-range force constants is common within the atomic Green's function formalism. More detailed analyses utilizing ab-initio techniques can be utilized for materials for which empirical potentials are not readily available. For the sodium chloride crystal used in this study, an empirical potential available from literature is utilized [76,77].

The novelty of this study is the inclusion of the long-range electromagnetic coupling across the gap, which allows for energy transfer at finite spacings. Therefore, the main focus on the

chapter will be the development of the force constants obtained from the long-range electromagnetic forces between ions. The forces will be obtained in a "first-principles" manner, in that the exact time-retarded Coulomb interaction between atoms will be calculated and summed with no phenomenological or macroscopic inputs. While the macroscopic Maxwell Equations require constitutive relations such as a dielectric constant characterizing the response of the system to imposed electric fields, the microscopic Maxwell Equations are utilized which give the exact description of electromagnetic fields for any number of atoms in vacuum. The microscopic Maxwell equations are solved to obtain the exact time-retarded fields emitted by the vibrating charged atoms of sodium and chloride, and summed up for all atoms in the crystal. The inclusion of both the short-range chemical bonding forces and the long-range electromagnetic forces between atoms allows for the calculation of transport both at contact and moving out to finite gaps.

2.1 Short-range force constants

2.1.1 Spring constants and phonon dispersion

Short-range force constants are electromagnetic in origin. When the electromagnetic forces between electron and nuclei of different atoms are calculated, the fields of these charged particles cause screening effects, therefore the overlap of the electronic clouds typically only extend to several neighbors of atoms. These complex microscopic effects of the individual electrons and nucleus result in a larger scale effect in which we are allowed to ignore the

intrinsic electron motion and employ what is known as the Born-Oppenheimer approximation [78]. For non-metals, the end result is that the nucleus and electron cloud of a given atom is treated as a combined particle since the relaxation of the electron cloud is at a much faster time scale. The interactions are given between atoms as spring constants, which stabilize the atoms into equilibrium positions on the lattice sites, and finite temperatures yield thermal fluctuations about the equilibrium lattice location. The most famous of these two-body interactions commonly employed in gas dynamics are the Lennard-Jones potential. Ignoring higher order multipole effects of the electromagnetic field [10], to lowest order the small shift of the electron cloud from the nucleus results in an electric dipole. The electromagnetic forces coupling these dipoles results in the Lennard-Jones potential of interaction between two neutrally charged atoms.

The atoms will space themselves in such a way so that they minimize their potential energy of interaction. At low temperatures, the dynamics of the atoms will be very small, i.e. close to the equilibrium lattice site locations. In this case, the lowest order Taylor expansion of the interatomic potential can be taken as a good approximation, for which the linear term in the spacing between atoms vanishes due to expansion about the minimum of the function. Therefore, Taylor expansion of the potential to lowest order results in a quadratic term. The quadratic terms in the potential energy yield linear forces of interaction between atoms, and these linearized forces are called the harmonic forces. They can be visualized as springs, which stabilize the atom by driving it back towards the equilibrium location whenever the atom shifts off

Before showing the short-range force constants for sodium chloride, we begin by first showing how the phonon dispersion (vibrational spectrum of the crystal) is derived from the

spring constants. The phonons are the lattice waves that transmit energy across an interface and

across the gap for subnanometer spacings. We first demonstrate the derivation for the simplest

crystal, a one-dimensional monoatomic chain to show the derivation of the vibration spectrum of

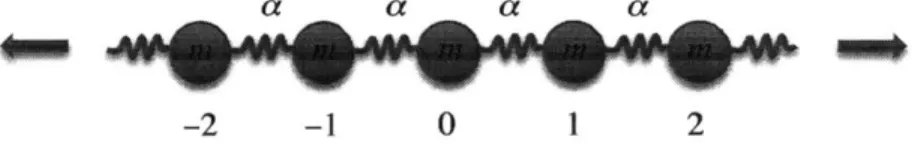
the crystal by utilizing the discrete Fourier transform. Suppose we have particles of mass m on

a crystal of sites separated by the distance a. The particles are connected by springs to their

nearest neighbor with spring constant a

.

Second nearest neighbor and higher order

contributions can be considered, but the mathematical approach is the same so we consider the

simplest scenario so as not to overcomplicate the derivation.

a

a

a

a

-2

-1

0

1

2

Figure 2-1: Schematic of monoatomic linear chain with nearest-neighbor coupling.

The dynamics of these atoms as given by Newton's

"d law is given by:

d

m T x, =a(x+ +xn_ ₁-2x,)

(2.1)

dt

In order to diagonalize the system, we utilize the discrete Fourier transform:

k( I= e-""lxn( (2.2)

Multiplying our dynamical equation by eib" and summing n over all integers yields the

diagonalized equation of motion:

-hk+4 sin2 k =0 _(2.3)

Equation (2.3) is exactly the dynamics of a single harmonic oscillator. The discrete Fourier transform has taken the coupled degrees of freedom of the atoms and transformed them from real space to the wavevector space where the dynamics are decoupled oscillators. By analogy to the single independent harmonic oscillator, we are able to recognize the frequency of the

monoatomic chain as a function of wavevector to be o, = 2 ja sin

().

dispersion is monotonic and consists of a single branch. For more complex models, say in the case of next nearest neighbor forces in the ID chain, it is possible to have non-monotonic

behavior [41].

0

k

a a a a

Figure 2-2: Monoatomic chain dispersion with non-monotonic behavior due to next nearest neighbor force effects. Figure adapted from Ref. [41].

A further sophistication, which is even more interesting and relevant for the sodium chloride

system, is the chain with a diatomic basis. In this case, an acoustic and optical dispersion branch is generated, yielding motion of the two-atom basis altogether (acoustic vibrations) or off phase vibrations of the two masses in a basis (optical vibrations).

(a) A(b)k

Sda 2xla 0 ,rI2b krb

kk

Figure 2-3: Dispersion of a diatomic chain. The dispersion shows a band gap for the case of mass mismatch (a) and the reduction to the monoatomic chain at the limit of equal masses (b).

Figure adapted from Ref. [41].

The optical modes are named as such due to their coupling to light and excitation due to electromagnetic waves. We will have more to say about this link between the electromagnetic fields and the coupling to acoustic and optical phonons in the chapters to come, but it suffices to say now that it has an important role in our understanding of thermal transport by conduction vs.

by radiation. In this section we have shown how lattice waves, i.e. phonons, are obtained from

the dynamics of the atoms. By considering springs coupling the atoms, the vibrational modes of the phonons are obtained. In the following section, the details of the short-range force constants are shown for the sodium chloride system.

2.1.2 Sodium chloride short-range force constants

For the short-range force constants of the sodium chloride crystal, we implement an empirical potential employed by Fuchs [76] and developed by Kellerman [77]. These short-range force

constants are the chemical bonding forces that hold the solid together and give rise to the vibration spectrum of the crystal.

A central potential is employed by Kellerman [77] with an artificial cutoff of nearest

neighbors and only depends on the separation between the two atoms. The Lennard Jones potential is also a central potential as it only depends on the separation distance between atoms. Fuchs et. al. [76] utilize this central potential and calculate the linearized forces that would results between the NaCl atoms through small vibrations about their equilibrium lattice sites. In the notation employed by Kellerman, more specifically, the central potential of interaction between two atoms is v(r) where r is the distance separating the two atoms [77]. Working in

the harmonic limit, the potential is expanded up to quadratic order,

v(r) = v(r)+v'(r)(r-rO)+Iv"(r)(r -r)2. The constant of the potential is not important as it

2

is removed when taking the derivative to calculate forces, but the first and second derivatives are needed to characterize the short-range force constants. The simple, elegant approach utilized by Kellerman is to ensure that the constants are chosen which ensure equilibrium for the atoms considering both a static Coulomb potential energy and the this short-range potential energy, as well as ensuring that the compressibility of the sodium chloride crystal is recovered. Defining first for simplicity two constants to represent the two derivatives of the central potential in nondimensional form, we have for the sodium chloride case [76,77]:

4r

s d

_v

dvr(2.4)

4r

_dv

Here e is the charge of an electron and r, is the lattice constant of the sodium chloride crystal. These two constants are obtained by utilizing two restrictions, the equilibrium condition of a given unit cell of the sodium chloride crystal and the ability to reproduce the bulk compressibility of sodium chloride. Considering the static Coulomb energy and the short-range potential energy due to the overlap of electron clouds, the energy in a given unit cell of sodium chloride can be expressed as:

2

(D(r) = -aM +6v(r) (2.5)

r

where r is taken to be the separation of the atoms, and this accounts for the interaction of a given atom with its 6 nearest neighbors. a. =1.7476 is Madelung's constant and accounts for

the approximation of treating the ions of the lattice as static point charges and summing over the entire lattice. The condition of equilibrium is such that the potential be a minimum at r = ro, so that we require D'(r) =0. The compressibility is directly related to the strength of the springs coupling the atoms, which is related to the second derivative of the potential energy by [77]:

1=-1D"(ro)

(2.6)

K 18ro

Utilizing the two conditions of equilibrium and compressibility, and the numerical values for sodium chloride of K =4.16 x 10 cm2 / dyne ,

ro

e =4.8 x 101 0e.s.u., the values for the constants A and B are obtained from Eq. (2.4). By expanding the force to linear order between every two atoms in the crystal, the force constants, (represented by v (r)) due to the short-range chemical bonding forces are generated [76]

The work here does not necessarily rely on empirical potentials in order to generate accurate results. The success of density functional theory (DFT) [72,79-82] in reproducing the force

constants of many systems from a first-principles makes it an attractive technique for crystals that do not have empirical potentials developed that accurately reproduce material properties. This focus of this study is the effect of the interplay between these short-range chemical bonding forces and the long-range electromagnetic forces for thermal transport at gaps near the contact limit. If the short-range force constants are able to generate the proper vibration spectrum of the system to be studied then it is accurate for the utilization of calculation of thermal transport. This is of course assuming a low temperature regime where anharmonicity is not important and

so a harmonic description is valid [64].

In the following section, the details for the electromagnetic force constants will be derived. It will begin with the fields emitted by a single vibrating charge, and using superposition the fields emitted by all charges can be calculated. The summation process for both the short-range force constants as well as the long-range force constants will be shown utilizing the notation of Fuchs et. al. to demonstrate the force constants that are inputted into the atomic Green's function

formalism [76].

2.2 Electromagnetic force constants

To obtain the force constants due to the electromagnetic forces, we look at the microscopic Maxwell equations, and derive the fields emitted by each atom. While inherently the origin of the short-range force constants are also electromagnetic in nature, the result of screening of the atoms yields a net force that only survives short distances as described in the introduction to this chapter. The net inclusion of the electromagnetic fields coupling atoms due to their monopole

charge terms for polar crystals as well as the dipole fields generated due to the intrinsic shift between the nucleus and electron cloud and the screening due to nearby atoms results in short ranged forces. In the contact limit, the short-range forces exist between the surface atoms and are stronger than the long ranged Coulomb forces. The long-range electromagnetic forces play a bigger role when a finite gap is introduced between the two materials. In this case, the forces between atoms across the gap are not screened, and only including the short-range forces up to a few nearest neighbors will artificially result in a predict conductance of zero without a force coupling the slabs across the gap. The model by Kellerman derived the harmonic force constants for the short range interactions by simply considering energy minimization and the ability to reproduce the compressibility of the crystal, as elaborated in the previous section [77]. There is a sense of a double counting that occurs when one considers both the short range force constants that derive from electromagnetic contributions as well from the atomic charge and intrinsic dipole as well as the long range electromagnetic monopole charge terms. This is typically alleviated by considering the Born effective charge for a crystal [78,83]. In this case, the true charge of the atoms used in the calculations is replaced with an effective charge that more accurately captures properties of the crystal. In this study, the charges taken were simply the electron charge and were not modified. The reasoning for this is the same reason why the empirical potential was employed as opposed to more accurate DFT models; the model was able to generate the vibration spectrum accurately and thus could be utilized in a Green's function

formalism [76].

To capture the long ranged behavior that yields interactions of atoms across a finite gap, the local fields are derived and the principle of superposition is utilized to capture the full physical picture. These force constants are "first-principles" in this sense, as the only information that is

required is the crystal lattice structure and the charges of the atoms to be able to construct the individual fields and perform the superposition. These force constants within the atomic formalism capture both the full effects of time-retardation, since the static Coulomb field limit is not taken, and nonlocal effects, as the simplification done in macroscopic electrodynamics for polar materials of a local dielectric constant depending only on frequency is not assumed.

For sodium chloride, the sodium and chloride atoms have a net positive and net negative charge of one electron, respectively. In this case, the lowest order electromagnetic fields emitted

by the system will be due to the monopole term. Higher order multipole effects [10] due to the

complex charge distribution of the nucleus and electron cloud are neglected. For the case of a neutral atom crystal, such as silicon or silicon dioxide as utilized in near field experiments [25,27,28], the atoms do not have a monopole contribution, and the lowest order effect is the dipole field, due to the intrinsic dipole of the atom because of the slight shift of the electron cloud from the nucleus of the atom. In the following section, the electromagnetic field of a moving point charge is derived in order to construct the force constants for the sodium chloride crystal. The principle of superposition is then utilized to obtain the net field within the crystal due to all of the individual fields emitted by the atoms [11].

2.2.1 Fields of single moving charge

We begin with Maxwell's equations in vacuum and will work in SI units to derive the exact time-retarded electromagnetic fields emitted by a single moving charge:

V-Z= p

80

VxE=-at

V

B=po J+"O

a

at

These equations are also known as the microscopic Maxwell equations, as the Maxwell's equations in the form given by Eq. (2.7) are valid for any charge distribution in vacuum. They do not assume an underlying medium within which the charges are moving and we can construct the charge distribution of a system by inputting the charges located at lattice sites, and as such they are microscopic. The equations are valid for a single charge, or for an infinite set of charges on a given lattice. As such, the material properties are those of vacuum, E = ro , Y=Y, and c is the speed of light in vacuum. This is because the equations do not assume an underlying medium, which is what the macroscopic Maxwell equations assume where the summation over atoms in the crystal has been performed under various simplifying assumptions [11,40].

The principle of superposition will allow us to first calculate the fields emitted by a single vibrating charge about its equilibrium lattice position, and sum the fields from all such atoms to obtain the total fields. The harmonic force constants, which include the short-range force constants as well as the long-range force constants, will yield small vibrations about the lattice position. By assuming motion of this type for the atoms, and calculating the fields emitted under such motion, the dynamics can be self consistently solved as will be shown in the next section [76]. The self consistent solving will yield the amplitude and frequency of the atomic vibrations that solves Newton's 2"d law for the dynamics of the motion when considering the

force constants of their mutual interactions due to short range forces as well as long range electromagnetic forces.

When the macroscopic Maxwell Equations are considered, an elaborate summation process is performed to sum over the effects of all of the atoms of the crystal [10,40]. The local approximation is often taken, characterizing the system's response to an external field as depending only on the frequency of the input light and not on the wavevector. More will be said about the macroscopic theory in Chapter 4 where the continuum model is calculated to compare with the atomic theory of this work. Here we proceed by deriving the solution to the microscopic Maxwell equations, which are the Maxwell equations for charges in vacuum with no assumed underlying medium the charges are bound to and we explicitly input the charges in the geometry of sodium chloride.

We derive the exact fields and calculate the force constants in order to be able to accurately calculate thermal transport when the sodium chloride surfaces are separated by sub-nanometer gaps. The second and third equations are automatically satisfied if the scalar and vector potentials are utilized, defined as:

E(,t)- _at

(2.8)

B(Ft)= V x A

We work in the Lorentz gauge, which relates the scalar and vector potentials:

i

A

1av =

_{V -A+--=0}

c2 at

(2.9)

where c is the speed of light in vacuum. The solutions to the vector and scalar potential differential equations are obtained as [11]: