Dispersion-managed solutions in the path-average normal dispersion regime

Dispersion-Managed Solitons in the Path-Average

Normal Dispersion Regime

by

Samuel Tin Bo Wong

Submitted to the Department of Electrical Engineering and Computer

Science

in partial fulfillment of the requirements for the degree of

Master of Engineering in Electrical Engineering and Computer Science

at the

MASSACHUSETTS INSTITUTE OF TECHNOLOGY

May 2001 O

®

Samuel Tin Bo Wong, MMI. All rights reserved.

The author hereby grants to MIT permission to reproduce and

distribute publicly paper and electronic copies of this thesis document

BARKER

in whole or in part.

_{in woleor}

_{n prt.OF}

MASSACHUSETTS _TECHNOLOGYINSTITUTE

JUL I120

LIBRARIES

Author . .. . ... .. .... . . . ...

Department of Electrica Engineering and Computer Science

May 23, 2001

Certified by ...

...

Hermann A. Haus

Institute Professor

Thesis Supervisor

C ertified by ...

..

...

...

Scott A. Hamilton

MIT Lincoln Laboratory Staff

4hes ,*Supervisor

A ccepted by ...

...

..

.

...

Arthur C. Smith

Chairman, Department'

Committee

on Graduate Students

Dispersion-Managed Solitons in the Path-Average Normal

Dispersion Regime

by

Samuel Tin Bo Wong

Submitted to the Department of Electrical Engineering and Computer Science on May 23, 2001, in partial fulfillment of the

requirements for the degree of

Master of Engineering in Electrical Engineering and Computer Science

Abstract

Optical fiber systems offer high-speed, broadband, and long-haul communications. Solitary waves known as optical solitons seem to be a natural means of transmitting data via fiber because of the balancing effects of linear anomalous group velocity dispersion (GVD) and nonlinear intensity-dependent self-phase modulation (SPM). Regular soliton systems (with uniform anomalous fiber), however, have yet to enter commercial markets because of high power requirements and timing jitter. Another class of solitary waves called dispersion-managed (DM) solitons, which can be man-ifested via a dispersion map, can resolve these issues. Numerical simulations and variational methods have shown that DM solitons can propagate in the path-average anomalous, normal, and zero dispersion regimes because of the interplay involving dispersion, nonlinearity, and chirp. In the net normal dispersion regime, a plot of pulse energy versus net dispersion yields two theoretical energy branches with map strength as a parameter. The lower-energy branch is interesting since it lies on a "quasi-linear" region near net zero dispersion. Having a net dispersion around zero means reduced timing jitter. The lower-energy DM solitons also need less power but exploit enough nonlinearity to fight GVD. So far, no one has claimed to have actu-ally found these pulses. This thesis provides preliminary experimental evidence for the existence of these lower-energy DM solitons. Numerical and experimental studies show a shifting of the transform-limited state position due to a dispersion imbalance. Under the proper initial launch conditions, nonlinearity can mitigate the effects of this dispersion-induced shifting in order to produce periodically stationary pulses. These DM solitons can potentially gain a edge over linear techniques used today. Thesis Supervisor: Hermann A. Haus

Title: Institute Professor

Thesis Supervisor: Scott A. Hamilton Title: MIT Lincoln Laboratory Staff

Acknowledgments

I would first like to thank Prof. Hermann Haus for his guidance and mentorship throughout my thesis project. I am forever grateful not only because he introduced me to an exciting area of research but also because he gave me some much-needed direction when my Lincoln group was suffering a mass exodus with people leaving for optical startup companies at that time. Like every one of his students before me, I am very honored to have Prof. Haus as my advisor. I would like to very much thank Scott Hamilton for acting as my Lincoln supervisor despite his having many other time-consuming duties in the Lab. I greatly appreciate his experimental advice and careful reading of my thesis. And, of course, I owe my skiing experiences to Scott.

There are also various other people I must thank. I thank Jeff Minch, my previous Lincoln supervisor, for showing me how to construct a recirculating fiber loop. I thank John Moores for answering my (naive) questions on dispersion-managed solitons when I first started to study them. I thank Prof. Erich Ippen for taking some of his valuable time to discuss my experimental setup and results and providing some very helpful insight (I wished I had more opportunities to talk with him). I thank Tom Murphy for his gracious help in the dispersion measurements and numerical simulations. I thank Leaf Jiang, my officemate on campus, for expressing interest in my thesis and for helping me on numerous occasions. I thank Bryan Robinson, the "Senior Staff" of the TDM lab, for giving me occasional experimental help and also Shelby Savage for letting me time-share the modelocked fiber laser (and providing some data for the laser). And I would like to thank Todd Ulmer for being cool and for introducing Van Halen to me so I can add punk, er, rock music to my "high-brow" repertoire of Bach, Beethoven, and Mozart (I need my daily dosage of "Hot for Teacher" to prep me up for labwork). Finally, I wish to extend my thanks to anyone in my Lincoln and campus research groups who had helped me in any little way.

I suppose at this point I need to give the obligatory thanks to my family like any acknowledgments section of a typical dissertation. Well, I'm not going to do that not because I don't care about them but because it seems so cliche. I know my family supports me no matter what endeavour I undertake and for me to openly thank them would be superfluous. Sincerity is all that matters. I would, however, like to take this opportunity to thank my support network of friends at MIT, especially those whom I met during my senior year. I guess I should also acknowledge someone at MIT (she knows who she is) for allowing me to realize that there's so much more to life than just math and physics. But I'm not going to talk about my philosophy on life right now. I shall reserve that for the acknowledgments section of my doctoral thesis.

Contents

1 Introduction

2 Background on Fiber Properties and Regular Solitons 2.1 Intrinsic Fiber Properties . . . .

2.1.1 D ispersion . . . . 2.1.2 Frequency Chirp . . . . 2.1.3 Nonlinearity . . . . 2.1.4 Attenuation . . . . 2.2 Wave Propagation in a Nonlinear Medium . . . . 2.3 Discussion on Regular Solitons . . . . 2.3.1 The Nonlinear Schrbdinger Equation . . . . 2.3.2 Properties of Regular Solitons . . . . 2.3.3 Limiting Factors for Regular Soliton Optical Networks

10 13 13 13 15 16 . . . . 19 . . . . 19 . . . . 22 . . . . 22 . . . . 26 . . . . 28

3 Background on Dispersion-Managed Solitons

3.1 Introduction to Dispersion-Managed Solitons . . . . 3.2 Methods for Theoretical Analysis . . . .

3.2.1 Numerical Simulation: The Split-Step Fourier Method 3.2.2 Approximate Method: The Variational Approach . . . 3.3 Behavior and Characteristics of DM Solitons . . . .

31 31 35 35 39 44 4 Experimental Search for Lower-Energy DM Solitons

4.1 Experimental Objective . . . .

48

4.2 Experimental Setup . . . . 4.2.1 Laser Source . . . . 4.2.2 Dispersion Map and Measurements . . . . 4.2.3 Other Measurements . . . . 4.2.4 Recirculating Fiber Loop . . . . 4.3 Experimental Results and Discussion . . . . 4.3.1 Preliminary Observed Effects of Power Level . . . . 4.3.2 Shifting of the Minimum Pulse Width Position . . . . . 4.3.3 Robustness of Pulses After Long-Distance Propagation 4.3.4 Achieving Periodically Stationary Pulses . . . . 4.3.5 Discussion of Experimental Results . . . . 5 Conclusion and Future Work

A Numerical Simulation of Experiments

. . . . 49 . . . . 50 . . . . 51 . . . . 57 . . . . 60 . . . . 61 . . . . 62 . . . . 66 . . . . 69 . . . . 70 . . . . 73 76 79

List of Figures

2-1 Diagram of anomalous group velocity dispersion and Kerr nonlinearity (self-phase modulation). Proper balancing between these two effects induces pulse shape stabilization for soliton propagation. . . . . 27 2-2 Comparison between NRZ and soliton transmission formats for the

given data stream . . . . . 30

3-1 Example of a symmetric two-stage dispersion map with a path-average anomalous dispersion . . . . 32 3-2 Numerical simulation of DM soliton in one unit cell of simple two-stage

dispersion m ap. . . . . 33 3-3 Variational plot of DM soliton energy versus net normal dispersion

with pulse width as a parameter (provided by Prof. Haus). The circles on the plot represent direct numerical simulations. . . . . 45 4-1 Autocorrelation (left) and optical spectrum (right) of the transmitter

laser pulse. The autocorrelation FWHM translates to At = 2.5 ps and the bandwidth tranlates to Av = 160 GHz for a time-bandwidth product of about 0.4, which is close to the Gaussian transform-limited state. The pedestal in the spectrum is ASE noise produced by an optical am plifier. . . . . 50 4-2 Experimental setup for dispersion measurements of fiber sections. . . 52 4-3 Group delay measurements (top) and dispersion calculation (bottom)

4-4 Group delay measurements (top) and dispersion calculations (bottom) for second anomalous segment consisting of 25 km AllWaveTM 55 4-5 Group delay measurements (top) and dispersion calculations (bottom)

for third anomalous segment consisting of 10 km SMF. . . . . 55 4-6 Group delay measurements (top) and dispersion calculations (bottom)

for normal segment consisting of 15 km DCF. . . . . 56 4-7 Group delay (top) and GVD (bottom) calculations for entire fiber loop

consisting of 75 km AllWaveT M _{, 10 km SMF, and 15 km DCF. Net} zero dispersion is achieved at 1550 nm as designed. . . . . 56 4-8 Experimental setup for EDFA transfer function measurements with

amplifier pump level varied between 1 and 10. . . . . 58 4-9 Measured amplifier gain and saturation power at different pump levels. 59 4-10 Experimental setup of recirculating fiber loop . . . . 60 4-11 Dispersion map with a tap at launch point located 7.5 km before the

anomalous half-cell center. . . . . 63 4-12 Experimental data of pulse width evolution versus loop period

mea-sured at the launch point in the fiber loop. . . . . 65 4-13 Illustration of how the tranform-limited state position shifts in the

linear case with negligible loss and nonlinear effects. . . . . 66 4-14 Autocorrelation of pulses after 3000 km propagation for "low" (left)

and "high" (right) power levels with 6.7 km SMF anomalous loop com-pensation fiber. . . . . 69 4-15 Dispersion map with launch point located 7.5 km after the anomalous

half-cell center and a tap located 1.7 km from the launch point. . . . 71

4-16 Experimental data of pulse width evolution versus loop period mea-sured at the loop tap point . . . . 72

A-1 Simulation of the linear case with only dispersion and no nonlinearity or loss... ... ... ... .81

A-2 Simulation of fiber loop with enough amplifier gain to compensate fiber lo ss. . . . . 8 2 A-3 Simulation of fiber loop with a higher pump level to show that

List of Tables

4.1 Measured loss for each fiber section in loop. . . . . 57 4.2 OTDR measured length for each fiber section in loop. . . . . 58

Chapter 1

Introduction

Optical fiber communications technology seems very promising in developing net-works that provide higher bandwidth, faster speeds, and more stable transmission of information. Essentially, optical fiber networks are the key to a better Internet. Issues exist, however, concerning the implementation of such systems due to the intrinsic physical properties of optical fiber. One major effect is dispersion, which causes tem-poral broadening of the optical pulse envelope as it propagates along fibers. Another well-known source of distortion is the Kerr-induced nonlinearity, which is significant if the intensity of the electric field of the optical pulse is sufficiently high.

Despite the inherent dispersive and nonlinear nature of optical fibers, it is possible to exploit these two apparently detrimental effects to create a stable, "particle-like" pulse, known as a soliton. Soliton systems use these fiber properties to their advantage

by compensating broadening due to dispersion with the compression imposed by

nonlinearity. Solitons seem to be ideally suited for the transmission of data in long-distance and high-speed communication systems.

However, despite the potential of solitons to balance nonlinearity and dispersion in fiber, no known commercial soliton system is currently deployed [1]. One of the primary reasons is that solitons suffer from jitter, a pulse-position modulation distor-tion that is dependent on dispersion and can be caused by a number of sources. The most well-known of such timing jitters is the Gordon-Haus effect, which results from amplifier noise. In addition, regular solitons exist only in the anomalous (negative)

dispersion regime. Propagation conditions require proportionality between soliton power and dispersion in the fiber. Solitons, therefore, cannot tolerate very low dis-persion (around zero disdis-persion) because the soliton power, along with the signal power, would vanish. This condition means that decreasing dispersion in order to reduce jitter comes at the expense of the signal-to-noise ratio. An additional dis-advantage for regular soliton systems is the high power required to support soliton propagation. High power levels are needed to induce sufficient nonlinearity to counter the high dispersion in optical fibers. In principle, regular solitons can exist with low energies if only fibers can be reliably manufactured with uniform low dispersion. This, however, is a fabrication problem because not only is it difficult to make fibers with low dispersion, fluctuations in the regime of low dispersion due to the manufacturing process may severely perturb the stable dynamics of a soliton pulse. From a com-mercial perspective, since linear techniques, whose philosophy is to fight or suppress nonlinearity as opposed to soliton methods, seem to perform quite well under the current circumstances (with data rates around 10 Gbit/s), there is no practical or desirable incentive to implement soliton systems by using higher power levels.

Dispersion-managed solitons address the issues described above and show promise in overcoming the limitations of regular solitons [1]. Dispersion-managed (DM) soli-tons occur in systems with fiber having spatially varying dispersion that is usually periodic. The advantages of such solitons over their conventional counterparts include enhanced energy, which leads to reduced Gordon-Haus jitter, and reduced pulse in-teraction, which leads to higher bandwidth efficiency [1]. Theoretical studies of fiber maps with segments of varying dispersion reveal that DM solitons can propagate at zero or normal (positive) average dispersion, whereas ordinary solitons propagat-ing through fiber of constant dispersion strictly operate in the anomalous dispersion regime.

The focus of this M.Eng. thesis is to investigate DM soliton propagation in the net normal dispersion regime near zero dispersion. Previous theoretical studies [2, 3, 4, 5, 6] have shown that two pulse energy solutions exist for a fixed net normal dispersion with pulse width and map strength as parameters, provided that the map

strength is sufficiently strong. The higher-energy solutions are not surprising since they are natural extensions of regular solitons; but, the lower-energy solutions are unique to DM soliton propagation in the net normal dispersion regime and have not been thoroughly investigated. The lower-energy DM solitons may have reduced optical power requirements comparable to systems employing linear techniques and so the implementation of DM solitons may be more attractive than regular solitons. Research on this "quasi-linear" lower energy branch may prove to be quite interesting if these lower-energy dispersion-managed solitons can be experimentally demonstrated to exist in the net normal dispersion regime.

The layout of this report is divided into five chapters. Chapter 2 gives brief background information necessary to understand the unique class of solitary waves called dispersion-managed solitons. In this chapter, various properties of optical fiber are discussed, along with the concept of the regular optical soliton. Chapter 3 provides some past preliminary theoretical and numerical studies on DM solitons in order to give motivation for this project. Chapter 4 presents the experimental work done for this thesis. The experimental setup, procedures, and results are discussed to demonstrate preliminary evidence for the existence of lower-energy DM solitary waves. Chapter 5 ends this dissertation with a conclusion and plans for future work.

Chapter 2

Background on Fiber Properties

and Regular Solitons

This chapter introduces some basic concepts that are helpful in understanding disper-sion-managed solitons. Wave propagation through fiber and material properties, such as dispersion, nonlinearity, and attenuation, will be discussed. A brief introduction to regular solitons, which requires constant anomalous dispersion, will be presented. A key tool discussed in this section is the Nonlinear Schr6dinger Equation (NLSE), which is one of the simplest ways to model nonlinear pulse propagation. A section on the implications of regular soliton implementation is also included.

2.1

Intrinsic Fiber Properties

2.1.1

Dispersion

The effect of dispersion on a pulse is temporal broadening. Consider the propagation constant (or wave number)

#

expanded in a Taylor series around an angular frequency

WO

1 1

where

#4 = , . (2.2)

The first two terms, #3 wo/v, and i1 = 1/v, in Eq. (2.1), are related to the phase (vp) and group (v_) velocities, respectively, and the third term

#2

represents group-velocity dispersion (GVD) or chromatic dispersion. GVD is the phenomenon of differ-ent frequencies (or wavelengths) in a pulse traveling at differdiffer-ent velocities. Since some optical frequencies under the pulse envelope propagate faster or slower than others, the envelope broadens in time as it moves along a dispersive medium. Dispersion is a linear phenomenon. According to the properties of Fourier transformation, a shorter pulse in time implies a larger bandwidth in frequency. Because wide-bandwidth pulses contain more frequency components traveling at different speeds due to dispersion, short pulses broaden at a faster rate than long pulses. GVD-induced intersymbol interference (ISI) is one challenge faced when implementing optical fiber networks employing ultrashort pulses.

GVD is often represented in terms of wavelength rather than in angular fre-quency. In terms of wavelength, the chromatic dispersion parameter is renamed to D = df31

/dA.

This definition can be related to

#2

in Eq. (2.1) using the dispersion

relation as follows

D=d 1 _D 27rc(23_{#2 .(2.3)} dA o( v9 A213

The minus sign in the equation comes from the inverse relationship between wave-length and angular frequency, as indicated in the expression Aw = 27rc. Note that the units for

/

is [ps2_{/km] while the units for D is [ps/nm -km]. The dispersion} parameter D can be physically interpreted as the spreading in time (in ps) per unit bandwidth of the pulse (in nm) over one kilometer of fiber.

There are two types of GVD: anomalous (or negative) and normal (or positive) dispersion. The type is determined by the sign of the dispersion parameter. A

negative 02 (or positive D) is defined as anomalous while a positive /2 (or negative D)

is normal. In anomalous dispersion, higher frequencies (shorter wavelengths) travel faster than lower frequencies (longer wavelengths). The converse is true in normal dispersion. In standard single-mode fiber (SMF), the zero-dispersion wavelength is approximately 1.3 pm with the anomalous dispersion regime spanning the longer wavelength side and the normal dispersion regime spanning the shorter wavelength side. Adjustment of the index of refraction profile and core dimensions in the fiber changes the zero dispersion wavelength and the dispersion slope, i.e. dispersion-shifted fiber (DSF) or dispersion-compensated fiber (DCF). To first order, an equal amount of anomalous dispersion completely compensates an equal amount of normal dispersion and this case is the simplest example of dispersion management.

Higher-order dispersions exist in addition to GVD. Third-order dispersion (TOD) or

/3

gives rise to a dispersion slope (i.e. how GVD changes over frequency or wave-length) and creates an asymmetry in the dispersion profile. Polarization-mode dis-persion (PMD) results from random polarization effects since fiber is a birefrigent material. To first order, two eigenstates exist where the fast principal axis is or-thogonal to the slow axis. When random polarization is induced on a propagating light pulse, different components of the wave fall onto one of the axes depending on their polarization. Since the components on different polarization axes travel at dif-ferent velocities, the end result is temporal broadening of the pulse envelope. GVD is generally the dominant dispersion component since its operational distance is the greatest. Higher-order dispersions, such as PMD, however, become non-negligible at higher transmission data rates.

2.1.2

Frequency Chirp

Frequency chirp refers to the time dependence of signal frequency. GVD induces linear chirp on an optical pulse propagating through fiber. Positive chirp occurs when the instantaneous frequency increases linearly from the leading to the trailing edge (also known as up-chirp) and for negative chirp, the converse is true. If a pulse is initially unchirped, its temporal width broadens by the same amount in either the anomalous

or normal dispersion regime. If a pulse is chirped, however, its behavior is different in different dispersion regimes depending on the sign of the chirp. A positively-chirped pulse temporally broadens in anomalous dispersion, but compresses initially followed by broadening in normal dispersion. Similarly, a negatively-chirped pulse broadens in normal dispersion, but compresses initially followed by broadening in anomalous dispersion. Chirped-pulse compression occurs because the GVD of one sign is temporarily compensated by a pulse chirp of the opposite sign. It can be inferred that anomalous dispersion generates positive chirp whereas normal dispersion induces negative chirp. If an optical pulse has no chirp, the time-bandwidth product

AwAt is minimized and the pulse is transform-limited.

2.1.3

Nonlinearity

Nonlinearity in optical fiber becomes a significant effect if the intensity of the electric field of the light pulse is sufficiently high. This fiber nonlinearity induces a change in

the index of refraction

n = no + n2JE12 , (2.4)

where no is the bulk material index and n2 is the new constant that determines how

the index increases with increasing optical intensity. This is known as Kerr-induced nonlinearity. The dipole moment per unit volume, or polarization P, can be written in a power series in terms of electric field E:

P = O(XN E + X(2)E2 + X(3)E+...) . (2.5)

co is the permittivity (or dielectric constant) in free-space and X(n) is an nth-order tensor representing the nonlinear optical susceptibility. Note that P and E are space, time, and frequency dependent. The first term in the series is the linear polarization

and the rest of the terms comprise the nonlinear polarization, that is,

PL =OX('E and PNL =EoX(2) E 2 + X(3)E3 +...) . (2.6)

In this analysis, only the dominant term in the nonlinear polarization is retained. For optical fiber, X(2) is negligible due to the inversion symmetry of the potential

around the SiO2 molecules. We can therefore describe the nonlinear polarization of

a propagating beam with angular frequency w in optical fiber as

PNL(W) = 3oXo( 3)(P : W, -w, w)E(w)12E(w) , (2.7)

where the coefficient of 3 accounts for the number of possible permutations for the electric field orientation (the degeneracy factor). The total polarization of the material system is now written as

Ptt = co(X(01E(w) + 3x(3 _{(W : w, -w, w)IE(w)|}2_{E(w)) = OXeffE(w) , (2.8)}

with the effective susceptibility defined as

Xef f = X(1 + 3X(3 (W : w, -w, w) IE(w) 12 . (2.9)

It is generally true that [7]

n 2 = 1 + Xef f (2.10)

and combined with the intensity-dependent index of refraction from Eq. (2.4), the linear and nonlinear indices can be related to the linear and nonlinear susceptibilities in the following manner:

and

n2 = .X (2.12)

8no

In a single optical fiber channel, the dominant Kerr nonlinearity described by X(3)

is self-phase modulation (SPM). SPM is a change in the phase of the optical pulse caused by the nonlinear index of refraction (a change in the index modifies the phase velocity). As described by its name, SPM is a modulation of the pulse phase due to its own intensity. This phase delay is proportional to the intensity of the pulse. The shift in frequency (Aw) is then the time derivative of the change in phase (Aq), i.e.

Aw- dt (2.13)

dt

Unlike linear processes, nonlinearity can modify the frequency content of a pulse and even generate new spectral components. In the case of SPM, frequencies are shifted up or down, depending on the sign of the nonlinearity. If n2 is positive, then the nonlinear index of refraction increases as the optical intensity increases. Since a larger index implies a smaller group velocity, the most intense portion of the pulse sees the most phase delay because of positive Kerr nonlinearity. In this case, Eq. (2.13) states that the "red" (longer wavelengths) portion of the pulse is shifted to the front while the "blue" (shorter wavelengths) portion is shifted to the back.

Other Kerr nonlinearities (third-order nonlinear processes) include cross-phase modulation (XPM) and four-wave mixing (FWM). XPM, present in multi-channel systems, involves phase modulation of a given pulsetrain channel by the intensity of an adjacent or orthogonal pulsetrain channel. FWM involves the interaction and energy exchange of pulses at two wavelengths that produce Stokes and anti-Stokes components at adjacent wavelengths if the phase conditions are matched. SPM and XPM are special cases of FWM.

2.1.4

Attenuation

No material is truly transparent and fiber is no exception. As optical pulses propa-gate through fiber, the intensity is attenuated due to material absorption and Rayleigh scattering. Silica in fiber absorbs in both the ultraviolent region and the far infrared region beyond 2 pm (hence, fiber loss is wavelength-dependent). Small amounts of impurities lead to absorption within a wavelength window, such as hydroxide ion implantation around 1400 nm during fabrication of single-mode fiber. Rayleigh scat-tering, intrinsic to all materials, arises when atomic dielectric fluctuations in the index of refraction scatter light in all directions. This scattering dominates at short wavelengths and sets the ultimate limit on fiber loss. Bending and splicing are also significant external contributors to fiber loss.

The attenuation constant a is typically used to describe fiber loss. If an optical pulse is launched with power P into a fiber of length L, then the transmitted power at the output is

Pt = Po exp(-aL) . (2.14)

The parameter a is conventionally expressed in units of dB/km and SMF commer-cially used today typically provides an attenuation constant of 0.2 dB/km at 1550 nm.

2.2

Wave Propagation in a Nonlinear Medium

In order to analyze nonlinear wave propagation, we start with Maxwell's equations. Consider Faraday and Ampere's Laws:

V x E =

_at

(2.15)

B9D

V x H = , (2.16)

where E is the electric field, H is the magnetic field, D is the electric flux, B is the magnetic flux, and J is the current density. Assuming an isotropic and uniformly magnetic medium with nonlinear polarization PNL, we define as constitutive relations

D=cE+PNL and B=pH , (2.17)

where E and /u are the permittivity and permeability, respectively. Note that E is the dielectric constant specific to the medium while y in this case is the free-space value, which can be denoted as po. Taking the curl of Faraday's Law and using J = c-E

(Ohm's Law) yield

E E _2E OPNL

V x V x E+ ou + tPoEt2 - - "

a

t2 (2.18)

Recalling that V x V x E = V - (V -E) - V2E and assuming V -E = 0 1 (no charge

density in Gauss' Law, which is the case for optical fiber), we obtain

a

a

2

_a2

PNL

( __E )E = /a

t2

(2.19)

From Eq. (2.19), we see that the nonlinear polarization acts as a source term to the classical Helmholtz equation. Considering the waves to be time-harmonic, i.e.

a/at

- -iw, we write

E(z, w, t) = 8 E(z, t)ei(kz-wt) (2.20)

and

PNL (Z, w, t) PNL(Z, t) ei (kpz-wt) (2.21)

where 6 and P are unit vectors and k and kp are the wave numbers in the direction of

the electric field E(z, t) and nonlinear polarization PNL (z, t), respectively. Substitu-'This is only an approximation for an anisotropic medium.

tion of these field definitions into the above wave equation yields

{02

O2+ 2ik - k 2) -E(z, t) ei(kz-wt)

We can simplify Eq.

/[t0, 2 a 1982 -

2iw)

-

22wt

- pOE

(at2

- 2iw a

at

- W2) . PPNL(Z, t)ei(kpz-wt) .(2.22)

(2.22): using the dispersion relation k2

= 1u₀Cw2 and applying

the slowly varying envelope approximation. This approximation states that many optical cycles are contained under the pulse envelope, that is,

OE a2E

k

>

Oz az2 OE

a

2_E

tat

at

2

OPNL W2_PNL

_at

(2.23) (2.24) (2.25) > 2PNL at 2

Eq. (2.22) now simplifies to

2 k+ POE-- E(z, t) = 2 ( - )PNL (Z, t)ei(kp-k)z

Recall that w/k = c/n where n is the index of refraction so that poE = (n/c)2 _and Eq. (2.26) becomes

OE(z,t) poo-c nEz____

+ E(z, t) + n E(zt)

Oz 2n c Ot

2i(oWC( -i)PNL (Z, t)e(kp k)z 2n

If we consider only the steady state and assume conductivity to be zero, which is realistic in optical fiber, the wave equation simplifies to

OE(z, t) Oz

SZOWC ')PNL(Z, t)ei(kp-k)z

2n (2.28)

Some general remarks:

1.) (kp - k) describes the difference in phase between the electric field and the nonlinear polarization.

(az

(2.26)

2.) If OE/Oz is real and PNL is imaginary, then there is growth or decay of the electric field.

3.) If OE/&z is imaginary (and PNL is real) and proportional to E, then the real part of the linear susceptibility is modulated and this induces a change in the phase velocity.

2.3

Discussion on Regular Solitons

The term soliton was used by Zabusky and Kruskal in 1964 when they described the particle-like behavior of numerical solutions solving the Korteweg deVries (KdV) equation [8]. These soliton solutions remain unchanged from collisions and interac-tions with one another and regain their asymptotic shapes, magnitude, and speeds. Hasegawa and Tappert theoretically showed in 1973 that an optical pulse in a di-electric fiber creates an envelope soliton and Mollenauer experimentaly demonstrated this phenomenon in 1980 [8]. These findings are perceived to be a breakthrough in optical fiber communications because short pulses distort after long-distance prop-agation due to dispersion in fiber. Proper balancing of nonlinearity and dispersion makes soliton pulse propagation possible over long distances in optical fiber.

This section starts with the derivation of the Nonlinear Schr6dinger Equation (NLSE), considered to be one of the most straightforward methods to model soliton behavior. The properties of solitons described by this equation will be presented and a discussion on implementation issues with solitons in fiber communication systems concludes the chapter. Note that we consider here only the case where the fiber has a uniform anomalous dispersion and these optical pulses, therefore, are generally called

regular solitons.

2.3.1

The Nonlinear Schrodinger Equation

We start with the slowly varying envelope wave equation in Eq. (2.28) describing propagation in a material with a nonlinear index of refraction and assume that the

electric field and nonlinear polarization vectors are aligned such that OE . NLOWC i(kp -k)z

Z =Z2n

Recall that the dominant nonlinear polarization term in optical fiber with a single wavelength channel is the third-order self-phase modulation (SPM), i.e.

PNL = CoX(3)|E|2

E CoX(3_{)EE*E , (2.30)}

which implies that

kp = k - k-+ k -+ k - k =0 - phase-matched . (2.31)

Thus, SPM is an inherently phase-matched process. By substituting Eq. (2.30) into Eq. (2.29), the wave equation becomes

aE ₊ n OE _{=2 -X} w-()1

. (2.32)

az C at 2cn

This is known as the nonlinear wave equation where the nonlinear term induces in the propagating solution a phase shift eicO that is proportional to the E-field intensity, i.e. q ~ X(3)E 12, as expected from SPM.

To model the propagation of the pulse envelope in optical fiber, we incorporate group velocity and dispersion into Eq. (2.32). For simplicity, we treat the Kerr nonlinearity independently from group-velocity dispersion and consider only the linear effects. If permittivity E(w) has a frequency dependence, so does the wave number

k(w) due to the dispersion relation. Following Eq. (2.1), we can approximate the

wave number as a Taylor expansion

Ok1 102 k

k(w) ~ k(wo) + (W - wo) + 2 (W - O) . (2.33)

Ow

W

2awo

its Fourier transform

E(z, t)e(k(wo)z-wot) -+ E(z, w - wo)ei(k(wo)z) (2.34)

where we used the Fourier transform property FT{x(t)e(wot)} < X(w - wo). For

small Az, the envelope must evolve as

OF

Az = (ik(w) - ik(wo))EAz , (2.35)

Oz

where k(w) is the actual phase and k(wo) is the assumed phase. This effectively corrects the envelope to consider the frequency dependence of k(w). By substituting Eq. (2.33) into Eq. (2.35), we obtain

aE

₌

Ak1

92 k

(W - WO) + (W _ wo)2

E

. (2.36)

Recall from Fourier transform theory that multiplication by -i(W - wo) in the fre-quency domain translates to a temporal derivative in the time domain. Using this property, Eq. (2.36) becomes

OE Ok OE 1 2_{k O}2_E

Z

Ow

WO -t 2 . (2.37)

Define 1/v9, Ok(wo)/Ow and k" = O2k(wo)/0w2 and add the nonlinear term from

Eq. (2.32) in Eq. (2.37) to obtain the nonlinear wave equation with group velocity,

dispersion, and nonlinearity:

OF + I OF= -k" 2 _{E ±-WX 3}

)lE12_E

. _(2.38)

Oz V9 Ot 2 Ot2 2cn

Note that k is generally assumed to be equivalent to

#

by convention, that is, k(w)

#(w)

2. The latter notation will be used throughout the rest of this dissertation. It 2

This is only an approximation since f is the longitudinal component of the wave vector k (we are considering only unidirectional propagation) and the transversal component is very small for the fundamental mode in a dielectric waveguide such as optical fiber.

is possible to eliminate the first-order time derivative in Eq. (2.38) using a change of variables. To represent the time parameter in the frame of the group velocity, we

rewrite the time variable as t -+ t - (9'__ )z = t - /3#z and Eq. (2.38) becomes

.u(z, t) /3" 02u(z, t)

2 z 2 at2 - yu(z,t)|2 u(zt) , (2.39)

where u(z, t) is the electric field envelope (replacing E(z, t) so that it will not generally

be confused as the electric field),

#3g

= 32 is the group velocity dispersion, and 7 = ( -n2)/(c - Aeff) is the Kerr nonlinearity coefficient with Aeff as the effective core area given by

f f If (x, y) 2dxdy

f f

jf(x, y) 4dxdy '

which is obtained by averaging the phase shift using the modal distribution profile f(x, y) over the fiber (integrating over tranverse directions x and y). Eq. (2.39) is the canonical form for the Nonlinear Schr6dinger Equation (NLSE), which is an integrable nonlinear partial differential equation frequently used to model optical soliton pulses. Note that this equation is adequate for pulses of width To > ips such that WOTO > 1.

Also, fiber loss quantified with the parameter a can be incorporated into the canonical NLSE. To model pulses as short as ~ 50 fs, however, a more generalized nonlinear Schr6dinger equation should be used [9]

OnU a .#2 a2u /3 1₃U

-u +2i- - -5

az 2 2 t2 6 t3 + ... [higher-order dispersion terms]

SZ uU2 + _Y 2 a(-uI2u) -TRU_RU

_(2.41)

1 WOa t

where a accounts for the intensity attenuation in fiber and TR (estimated to be

~ 5 fs) is related to the slope of the Raman gain. Note that in addition to higher-order dispersion (TOD, PMD, etc.), Eq. (2.41) also includes higher-higher-order nonlinear effects such as stimulated inelastic scattering (Raman and Brillouin gain) in addition to Kerr nonlinearites (SPM, XPM, FWM).

2.3.2

Properties of Regular Solitons

Analytic solutions have been found for the NLSE via the inverse scattering method [10], which maps the solution of the nonlinear PDE to solutions of linear differential equations solvable by standard methods. If /2 < 0 (i.e. anomalous GVD) in Eq. (2.39), the lowest order (N = 1) solitary wave solution, or the fundamental soliton, has a hyberbolic secant profile [11]:

t - #2AWOZ

U(z, t) = Ao sech ) exp (-iAwot)

x exp

[i

( + 12Aw2) z] exp (iq) , (2.42)

with the constraint that the parameters T and AO obey

1 -IAoI 2

(2.43)

r2 02

In Eq. (2.42), AO is the amplitude, T is the pulse width,

4

is the phase, and AwO is the

detuning from the nominal carrier frequency wo. If there is no detuning, the profile is a simple sech function of amplitude AO with an accumulated phase delay of -YIAo I2_z/2

due to the Kerr effect from the average intensity. If there is detuning by AwO, the propagation constant changes by

#2Aw/2

in the phase factor and the inverse group velocity changes by 32Awo, which produces a timing shift in the argument of the hyperbolic secant. Soliton solutions of the NLSE obey the area theorem [11] where the area of the amplitude is fixed, i.e.

Area = ju(z, t)Idt = r . (2.44)

Hence, the energy of the soliton is inversely proportional to the pulse width T. The area theorem stipulates the amount of energy required for soliton propagation.

Higher order solitons solutions (N > 1 E integers) also exist for the NLSE. They are obtained if the pulse width is kept fixed and the amplitude takes on values that are integer multiples of the fundamental soliton amplitude as defined in Eq. (2.43) [12].

Such pulses exhibit more complicated oscillatory behavior that involves pulse breath-ing (compression/decompression) and pulse splittbreath-ing before returnbreath-ing to the inital higher order soliton waveform. Because of the inherent difficulty in implementing N-solitons, only the fundamental (N = 1) soliton is considered in this paper.

In the canonical form of the NLSE as shown in Eq. (2.39), an obvious interplay exists between dispersion (denoted by

#2)

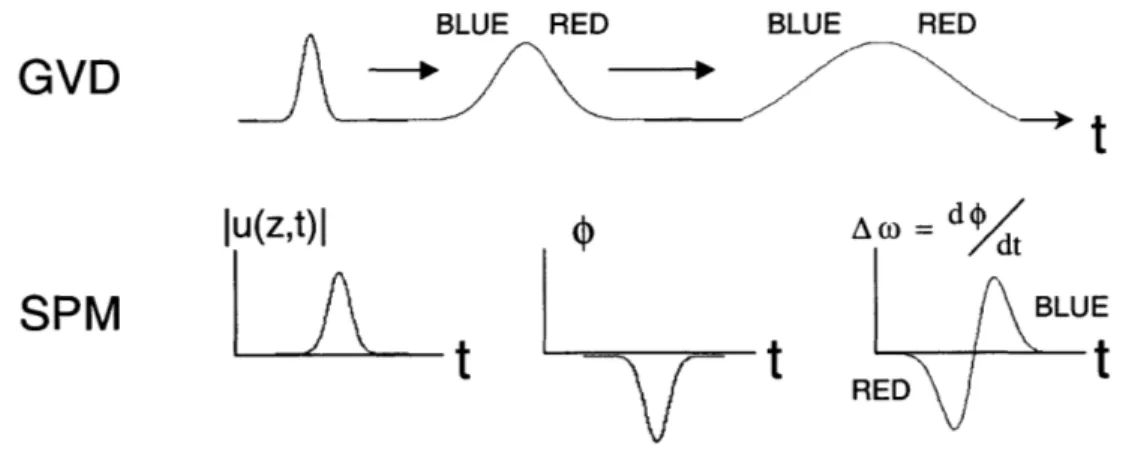
and nonlinearity (indicated by 7) in the evolution of the pulse envelope u(z, t) as it propagates along distance z. The nonlin-earity compensates for the dispersion and creates its own potential well [11]. Recall that Eq. (2.42) is only a solution to the NLSE when the GVD in fiber is anomalous (/32 < 0) and the blue light (shorter wavelengths) travels faster than the red light (longer wavelengths). This anomalous dispersion counter-balances the Kerr effect that shifts the red light forward and pushes the blue light backward. Fig. (2-1) shows an illustration of the compensation between dispersion and nonlinearity for soliton propagation in optical fiber. Note that while the shape of the optical pulse envelope is maintained due to the balance of anomalous GVD and SPM, it is not exactly the same pulse because of a resultant nonlinear phase shift induced by the nonlinearity. If the GVD is normal (/2 > 0), severe temporal broadening results because both phenomena reinforce each other.

BLUE RED BLUE RED

GVD

t

Iu(z,t)I

Aw = d t

SPM

BLUE

RED

Figure 2-1: Diagram of anomalous group velocity dispersion and Kerr nonlinearity (self-phase modulation). Proper balancing between these two effects induces pulse shape stabilization for soliton propagation.

Because of the counter-balancing effect between anomalous dispersion and Kerr nonlinearity, an optical soliton remains stable even for long propagation distances. If an arbitrary (non-pathological) pulse is launched into the fiber, it eventually evolves into a steady-state soliton pulse by shedding a continuum of dispersive waves in its transient stages. The term soliton inherits its name because of its particle-like behav-ior. Strictly speaking, a solitary wave, which is a solution to a class of mathematical equations to which the NLSE belongs, is only a soliton if it emerges unscathed from a collision or pulse-to-pulse interaction [11, 13]. While two solitons colliding into each other experience some timing and phase shifts, they both fully recover their pulse shape and energy. Surviving adjacent pulse collisions is desirable in multi-channel optical systems.

2.3.3

Limiting Factors for Regular Soliton Optical Networks

Regular solitons, so-called because they require constant dispersion along the entire fiber transmission line, seem like the ideal choice for reliable ultrafast, broadband, and long-haul propagation. They have implementation limitations, however, that prevent them from being the silver bullet of optical fiber communications. These issues include careful fiber plant physical layout, pulse distortions more pronounced in regular soliton systems, and undesirable high power requirements.

One possible drawback of regular solitons is that they require constant (anoma-lous) dispersion. As a result, physical implementation issues exist. The creation of a long link of fiber that has uniform dispersion is impractical for multi-channel sys-tems because each wavelength sees a different GVD and it is not possible to maintain constant dispersion over a wide range of wavelengths. In addition, fiber cables previ-ously installed underground already do not contain anomalous dispersive fiber with constant GVD.

A more serious obstacle to using solitons in optical fiber networks is the impair-ment caused by spontaneous noise in erbium-doped fiber amplifiers (EDFA). Since fiber is intrinsically lossy, long-distance pulse propagation requires signal amplifica-tion via some gain medium, such as an EDFA, in order to compensate for the fiber

loss. These optical amplifiers, however, introduce amplified spontaneous emission (ASE) noise. This effect degrades the signal-to-noise ratio (SNR) at the receiver and causes random jitter in pulse arrival times. This timing jitter is known as the Gordon-Haus effect [14]. At each optical amplifier in a transmission fiber system, ASE adds a certain number of photons per mode of white noise to the signal. This leads a small random change to the central frequency of each pulse. Because of group velocity dispersion, the random walk experienced by the carrier frequency results in a pulse-position perturbation, i.e. timing jitter. The variance of the timing changes (note that the mean is zero assuming the effect is modeled as a white-noise stochastic process) is expressed as [4, 14]

(At2) 1.76hwOynsp I A 2|(G - 1)z

3 _{ESO1 (2.45)}

9

TFWHMLa E

with h as Planck's constant, wo as the carrier frequency, n, as the ASE coefficient, G as the gain, La is the amplifier spacing, TFWHM is the pulse width at full-width half-maximum, and E,01 is the soliton energy. Notice that the timing fluctuations increase with distance cubed and so the Gordon-Haus effect typically dominates after long-haul propagation. For high data rate communication systems, randomly dis-placed pulses can end up in neighboring slots and thus cause detection errors at the receiver. In Eq. (2.45), timing jitter is proportional to GVD. So one way of reducing timing jitter is to decrease dispersion. While Eq. (2.45) predicts that zero dispersion completely eliminates jitter, solitons require a finite amount of dispersion for them to exist because the soliton energy is proportional to the dispersion from Eqs. (2.43) and (2.44). If the dispersion is very small, then the soliton has very small energy, which compromises the SNR. There has been much research on reducing timing jitter via other means such as narrow-band filters (passive methods) and in-line synchronous modulators (active methods). Methods involving the sliding guiding filter principle [4, 15], however, are undesirable to system engineers because this requires different filters in subsequent amplifier pods to slowly guide the pulse spectrum away from the ASE noise.

Another major implementation issue involved with regular solitons is the require-ment for high optical power levels. This is primarily due to the fabrication constraint where fibers with uniformly low dispersion is very difficult to manufactured. In order for the SPM to balance GVD, the intensity of the optical field must be high enough to cause a change in the index of refraction. Typically, the power and energy levels of regular solitons are quite high in order to induce the required SPM magnitude since the nonlinear index coefficient n2 is relatively weak. Such high power requirements

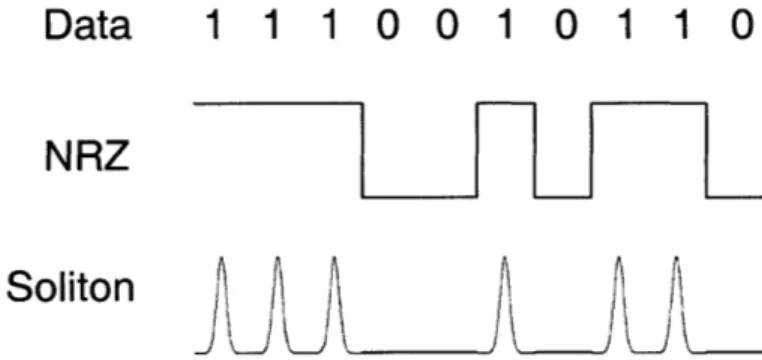
are inefficient, impractical, and often unacceptable in communication networks. As an aside, soliton systems have yet to win a competitive edge over linear tech-niques currently employed in the market. These "linear" techtech-niques counter the philosophy of soliton propagation by suppressing or minimizing the Kerr nonlinearity instead of exploiting it. One example is nonreturn-to-zero (NRZ) pulse transmission. This modulation format consists of rectangular ones (pulse train of bits) forming a continuous block if two or more ones ("1") occur consecutively and falling to null if a zero ("0") occurs. Fig. (2-2) provides a comparison between NRZ and soliton pulses. At today's 10 Gbit/s data rates, these linear techniques work remarkably well and

Data

1

1

1 0 0 1

0 1

1

0

NRZ

Soliton

Figure 2-2: Comparison between NRZ and soliton transmission formats for the given data stream.

have been implemented commercially. They also have power/energy levels far below those required for regular soliton communications. For tomorrow's higher 40+ Gbit/s data, however, soliton-based optical systems may be required to manage higher order effects such as PMD and to provide spectral efficiency.

Chapter 3

Background on

Dispersion-Managed

Solitons

This chapter describes a new class of optical solitary waves called dispersion-managed (DM) solitons. The dispersion map, which is the basic structure in creating such pulses, is discussed, along with the dynamics and evolution of DM solitons. Some of the underlying physics is revealed via numerical simulations and variational methods. Finally, properties of DM solitons are presented in comparison to regular solitons in order to provide some motivation for this thesis.

3.1

Introduction to Dispersion-Managed Solitons

A new class of solitons called dispersion-managed solitons can be created using disper-sion management [16]. Disperdisper-sion management is a technique that uses varying dis-persions, rather than uniform dispersion, in a link of fiber. Dispersion compensation occurs when one fiber segment of a particular dispersion is used to counter-balance the pulse broadening in another segment of opposite dispersion. A DM soliton is manifested on a fiber with a dispersion map, which consists periodic segments of dis-persion of alternating signs. Usually, these periodic segment structures, or unit cells, are placed one after another to produce periodic dispersion compensation in the fiber link. An example of a dispersion map is depicted in Fig. (3-1).

Unit Cell

Norm

t>

Anom _

rve

Figure 3-1: Example of a symmetric two-stage dispersion map with a path-average anomalous dispersion.

The simplest dispersion map is constructed from two fiber segments, one anomalous and the other normal. The lengths of the segments can differ. The path-average (net) dispersion associated with a particular map is given by [16]

- _ #"L , +/ La (3 1)

ave - L, + La '

#" and Lj refer to the jth segment's dispersion and length, respectively. For consis-tency throughout this section, parameters with the subscript 'n' correspond to the normal dispersion fiber and those with the subscript 'a' correspond to the anoma-lous dispersion fiber. Another characteristic of a dispersion map is the map strength, defined as

S_ | (#'Ln - 0"La)| _(3.2)(32

FWHM

where TFWHM is the minimum full width at half maximum (FWHM) of the pulse when

unchirped. The map strength represents a single dimensionless quantity that mea-sures the difference between the total dispersion accumulated in both fiber segments, rather than the difference of the two dispersion values. Essentially, S measures the

spreading factor of the pulses. Higher magnitudes in either segment lead to stronger maps since a larger dispersion swing spreads the pulses more. Pulses with shorter pulse durations also disperse quicker. Shorter pulse widths therefore effectively lead to higher map strengths.

Within each period of the dispersion map, the pulse undergoes breathing, which is marked by compressing and broadening in time [16]. Unlike regular solitons, DM solitons are not continually stationary but periodically stationary. The pulse returns to its original pulse shape after each map period (a unit cell). A numerical simulation of a DM soliton traveling through a unit cell of a dispersion map is shown in Fig. (3-2). The DM soliton temporally broadens and compresses twice within each map period and the chirp in each of the fiber segments has opposite signs. If power loss is neglected and the nonlinear coefficient is assumed to be the same for both fiber segments, then the pulse is narrowest, and hence unchirped, in the middle of each fiber segment. The pulse is also broadest and most strongly chirped at the boundaries between the anomalous and normal fiber segments.

0-W

Distance _Time

Figure 3-2: Numerical simulation of DM soliton in one unit cell of simple two-stage dispersion map.

the coupling of energy from the launched pulse into the soliton and to minimize the generation of dispersive radiation. In the dispersion map, the pulse experiences periodically varying chirp during propagation through each unit cell. If the optical source used to generate short pulses is chirped, the source must be located at a position in the unit cell where the chirp of the source matches that in the unit cell in order to maximize DM soliton coupling efficiency [16]. If the source is assumed to be transform-limited and generating unchirped pulses, the dispersion map should begin at the mid-point of one of the fiber segments in the unit cell where steady-state pulses are chirp-free. This argument provides the reasoning for implementing the first unit cell in a dispersion map with half the length of the anomalous (or normal) dispersion segment used in subsequent two-stage unit cells in the dispersion map. If this map starts with the full length rather than half-length and the launched pulse is unchirped, then there is a large transient response that sheds energy into dispersive waves until the pulse reaches the steady-state where it is chirp-free at the center of subsequent fiber segments in the map. Ideally, a pulse can be launched anywhere on the dispersion map period, provided that the chirp of the source is appropriately chosen for the launch location in the map. If there is gain (or attenuation) in the dispersion map, then the chirp-free point is not in the center of either segment anymore. In this case, the chirp of the source can be adjusted in order to move the unchirped location to the middle of the anomalous and normal fiber sections in a two-stage dispersion map. A feature that differentiates a DM soliton from an ordinary soliton is that solitary wave propagation in dispersion maps does not assume stable pulse shapes that are hyperbolic secant [16]. As map strength S becomes stronger, the shape changes from a sech-profile to a Gaussian. The time-bandwidth product increases from 0.32 (sech) to 0.44 (Gaussian). When the map becomes even stronger, pulses may assume shapes having even higher time-bandwidth products [16].

3.2

Methods for Theoretical Analysis

Numerous theoretical studies have been done on dispersion-managed solitons in order to shed some physical insight on such optical pulses. While numerical simulations of pulse propagation determined by the NLSE yield accurate physical predictions, the solutions are not analytic since the addition of dispersion management renders the NLSE non-integrable and the trial-and-error process of selecting parameters is tedious. Although such techniques do not rigorously describe the details of pulse propagation, approximation methods can be used to generate analytic solutions, which are typically a set of coupled ordinary differential equations. The two most frequently employed methods for soliton numerical modeling are the split-step Fourier method (rigorous numerical simulation) [9] and the variational approach (approximate analytic tech-nique) [12] and each of these is discussed in the following sections. Note that these algorithms were initially applied to regular soliton transmission and then extended to describe the propagation of DM solitons.

3.2.1

Numerical Simulation: The Split-Step Fourier Method

The most popular and perhaps most efficient numerical algorithm in solving the NLSE is the split-step Fourier method [9]. This technique uses the Fast Fourier Transform (FFT) to propagate the waveform through dispersive fiber in the absence of nonlinearity and treats the nonlinearity as a lumped element between the steps. Since the split-step algorithm uses the FFT (which scales by N log N, where N is the number of operations), the relative speed of this method is approximately one order of magnitude faster than finite-difference methods in achieving comparable accuracy [9]. The computational efficiency of the split-step Fourier method is thus one of the reasons for its popular usage in simulating pulse propagation in nonlinear dispersive medium.

(2.39) in the form

u=(D+N)u (3.3)

where D is the differential operator accounting for dispersion (and also absorption) in a linear medium and N is the nonlinear operator accounting for the effect of fiber nonlinearities. These operators are defined as

#'/2 &2

1a\

D = -_

a-

(3.4)

2 at 2 '

N

=_ Z'yn2 . (3.5)

Note that the term inside the parentheses in the linear operator b accounts for the loss. While the dispersive and nonlinear effects typically act on an optical pulse simul-taneously during propagation through fiber, the split-step Fourier method generates an approximate solution by propagating a small distance step size f and assuming that the linear and nonlinear effects operate independently. In the simplest case, the propagation from z to z + t can be executed in two steps, the first where disper-sion acts alone (N = 0) and the second where nonlinearity acts without dispersion (D = 0). The mathematical solution to Eq. (3.3) is [9]

u(z + f, t) ~ exp(Ne) exp(Th)u(z, t) . (3.6)

The dispersive effect can be solved within the Fourier domain (since dispersion is linear). If we take the Fourier transform of the linear part of Eq. (3.3), i.e. consider only the

b

operator, we have

(9u _{-Z =t2} #2

U2

~U./3T a~

Z

-> = - 2,U U (3.7)