Zhang-Zagier heights of perturbed polynomials

C

HRISTOPHE

D

OCHE

Zhang-Zagier heights of perturbed polynomials

Journal de Théorie des Nombres de Bordeaux, tome

13, n

o

_{1 (2001),}

p. 103-110

<http://www.numdam.org/item?id=JTNB_2001__13_1_103_0>

© Université Bordeaux 1, 2001, tous droits réservés.

L’accès aux archives de la revue « Journal de Théorie des Nombres de Bordeaux » (http://jtnb.cedram.org/) implique l’accord avec les condi-tions générales d’utilisation (http://www.numdam.org/conditions). Toute uti-lisation commerciale ou impression systématique est constitutive d’une infraction pénale. Toute copie ou impression de ce fichier doit conte-nir la présente mention de copyright.

Article numérisé dans le cadre du programme Numérisation de documents anciens mathématiques

Zhang-Zagier heights

of

_perturbed

_polynomials

par CHRISTOPHE DOCHE

RÉSUMÉ. Dans un

_précédent

_article,

nous étudions le _spectre de la hauteur de

_Zhang-Zagier

_[2].

Les

_progrès

_{accomplis reposaient}

sur un

algorithme

_qui

donnaient des

polynômes

_possédant

une pe-tite hauteur.

Ici,

nous décrivons un nouvel

_algorithme

_qui

_produit

des hauteurs encore

_{plus petites.}

Ceci nous a _permis de mettre

en évidence un

_point

d’accumulation inférieur à

_1,289735.

Cette

borne est meilleure _que la

_{précédente qui}

était

_1,2916674.

_Après

quelques

définitions nous détaillons le

_principe

de

_{l’algorithme,}

les résultats obtenus et la construction

_{explicite qui}

mène à cette

nouvelle borne.

ABSTRACT. In a _previous article we studied the _spectrum of the

Zhang-Zagier height

[2].

The _progress we made stood on an

al-gorithm

that

_produced

_polynomials

with a small

_height.

In this

paper we describe a new

_algorithm

that

_provides

even smaller

heights.

It allows us to find a limit

_point

less than 1.289735

i.e. better than the

_previous

one,

namely

1.2916674. After some definitions we detail the

_principle

of the

_algorithm,

the results it

gives

and the construction that leads to this new limit

_point.

1. Introduction

Let P be a

_polynomial

in

7~ ~zl, ... , zn~ .

The Mahler measure of P is then

defined

_by

If P is a one variable

polynomial,

ensures that

, Jensen’s formula

In this case we denote the absolute Mahler measure of P i. e.

M(p)l/d

_by

If a E

_Q,

we _{agree that}

M(a)

and

_represent

_respectively

the Mahler measure and the absolute Mahler measure of the irreducible

polynomial

of a with coefficients in Z. The

Zhang-Zagier height

or

_simply

the

_height

_{of a,}

denoted

_by

_ij(a),

is then defined as

ij(a) =

From results of

_Zhang

and of

_Zagier

_(cf.

_{(10~, [9]),}

we know that if a is an

algebraic

number different from the roots of

_{(z2 - z)(z2 - z}

+

_1),

In fact

_Zagier

_gives

also the

_algebraic

numbers which lead to an

equal-ity

in

_(3).

_They

are

exactly

the roots of

_z)

where

represents

the 10th

_{cyclotomic polynomial.}

Our contribution to the

_study

of the

_{Zhang-Zagier height}

consisted

_firstly

of an

improvement

of

(3),

namely

"

Theorem A. Let a be an

algebraic

number. We _suppose that a is

different

from

the roots

_of

(z2 - z)(z 2 _z

+

_z).

Then

Secondly,

we

proved

that V =

( a

_E

Q}

admitted _a limit

point

less

than 1.2916674.

_Finally,

we discovered an

_{algebraic integer}

whose

_height,

1.2875274 ... ,

is less than the smallest

_previously

known i. e. 1.2903349 ...

[4].

All this work is described in

_[2].

From

_this,

we are able to do a little better with the

_help

of a new

algo-rithm. In fact the main result of this paper is the

following.

Theorem. The smallest limit

_point

_of

V =

I

a E

_Q}

is less than 1.289735.

Before we _prove this

theorem,

we

display

some

_strange

relations.

Let

These

_polynomials

are remarkable

according

to their

height.

Indeed

A,

and

_A2

have a trivial

_height,

§(A3)

is the second

point

of the

_spectrum,

the smallest knomn

_height

_greater

than 1.2720196 ... We

_verify

that

So we make the

hypothesis

that small

heights

come from

_"perturbed"

poly-nomials of small

_height.

The

_phenomenon

seems

_{quite general}

since it occurs also for the Mahler

measure

[6]

and for the

_spectrum

of

a))ajt(l - 1/a) [3].

Therefore it is

_quite

reasonable to test

_polynomials

_A6(Z)

such that

with the

_ai’s

in N

_verifying

al + a2 +

2a3

+

4(a4

+

a5)

+

8(a6

+

a7)

31.

The results are

quite

disappointing

since we

_get

_only

a handful of

_good

polynomials;

the best

_{corresponding}

to the choice

having

fj(A6)

= 1.2906235... We also notice that

As(z)

is invariant under

the map z H 1- z.

_Now,

_every

_polynomial

of even

_{degree symmetric}

under z e 1 - z can be

expressed

in terms of

For instance

_z)

=

X4 - 2X3

₊

4X2 -

3X ₊ 1.

After _many unfruitful

_computations

we decided to consider

_only

polyno-mials in the new variable X and no

longer

in z. As a _consequence we can

Then we choose a

_{starting polynomial}

P(X)

of

degree d,

we take

_integers

and ask

_simply

that

9

At this

_point,

for each combination of

_verifying

₍₄₎

we estimate

by

the method of GraefRe

_[1].

If this evaluation is rather small we

_compute

precisely

its

_{Zhang-Zagier height.}

Before we did this

search,

we knew

only

9

_polynomials

whose

_height

is less than 1.29.

_Now,

_just

for the

_degree

28

we have more than 120

_polynomials

with a

_height

less than 1.29.

_Figures

1 and 2

_help

us to see the _gap we filled.

FIGURE 1. Known

_heights

_previously.

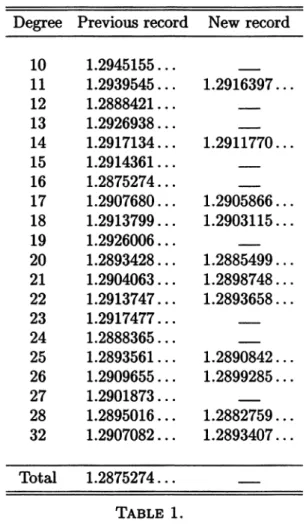
Table 1 shows the

_improvements

_made,

if _any, for each

_degree.

TABLE 1.

It is hard to

_{explain precisely why}

this

_{algorithm gives good}

results. Nevertheless here is a kind of heuristic

_argument.

In

[2],

we observed the

importance

of the resultant for the

_Zhang-Zagier

_height.

_Namely,

a

poly-nomial P with

_{sj (P)}

small

_usually

has a small resultant with each of the

Pi.

Now it is obvious that

for _any

_Pi.

So P ± are

_good

candidates.

These results convince us of the existence of a limit

point

of V less than

1.29 which leads us to the Theorem. To prove

it,

we use the same

_techniques

as in

~2~ .

2. Proof of the Theorem

With the above

_notations,

we shall use

Pl (X ), P2(X), P4(X), P6(X)

and We introduce also

_Q(X)

= with

and

We

_verify

that

Finally,

we need the

following

lemma

proved

in

[2].

Lemma. Let P be a

pol ynomial in

two

variables y

and _z, such that

degzP

&#x3E; 0.

_{Let (n}

be and assume that

for

all n and all

k,

(P9

z) is

not

_identically

zero. We then have

At

_present

let

_(ql,

q2, ... ,

q5) E

Q~

and b a denominator of the Then it is clear that

gives

rise to a limit

_point

of

I

P E when n tends to

_infinity.

tends to

_infinity

is

_equal

to

As the

_degree

in z of the

_polynomial

in the

_product

of

₍₆₎

is

the Lemma asserts that

₍₆₎

_equals

Now recall

₍₁₎

and

_{put ;}

and

_{finally by}

b

_changes

of variable t N t

_{+ j/b,}

_{for j}

E

_[1,6],

we

_get

The trick of

_{considering z}

as a

_simple

_parameter

_together

with formula

(2)

imply

that

₍₈₎

is

_nothing

but

where

_log+(w)

is

_max(0,

_log

_{I w 1)}

as usual. Then we search the best

qi’s

in

order to have the smallest limit

_point

_possible.

As we can

_easily

_compute

the

_simple

_integral

of

₍₉₎

_by

a Riemann _sum, we test several choices of

_qis.

Thanks to these

_{computations,}

we

_produce

a limit

_point

less than 1.29 thus

better than the

_previously

known i. e. 1.2916674. The best combination found is

which

_yields

a limit

point

less than i = 1.289735. Of course this limit

_point

i concerns

only polynomials

but

fortunately

we can deduce from them a

subsequence

of irreducible

_polynomials

with a

_height

less than

_(see

_[2,

Let us _say a few words about the

_polynomials

involved in

(9).

While

the selection of

_Pl,

_P2,

_{P4, Ps,}

_P8

_among

_{Pl, ... , Ps}

is forced

_by

the search of the minimum of

_(9),

the choice of the

_polynomials

to be

_perturbed

is

more

_arbitrary.

In

_fact,

we take

_Qi(X)

and

_Q2(X)

_according

to their

very small

height

and also because we remarked that a

_{product of Pi’s,}

namely

divides

_{Qi (X) - Q2(X).}

This

_point

seems

determinant,

however we do not understand

_why

it is so

important.

The results confirm that our

_previous

_algorithm

was far from

_being

ex-haustive since a

_great

number of

heights

were missed.

Nevertheless,

no new

height

less than 1.2875274 appears, so that we still do not know the second non-trivial

_point,

if any, of V. It would be desirable to

improve

our

knowledge

of the set

_{of S5 (a)}

as it was done for the Mahler measure of

non-reciprocal polynomials

[7]

and for the

_height

of

_totally

real

_algebraic

integers

[8]

and

_(5],

but we have the

_feeling

that this will be difficult without new ideas.

References

[1] J. _DÉGOT, J.-C. _HOHL, O. _JENVRIN, Calcul _numérique de la mesure de Mahler d’un poly-nome par itérations de Graeffe. C.R. Acad. Sci. Paris 320 _(1995), 269-272.

[2] C. _DOCHE, On the _{spectrum of} the _{Zhang-Zagier height.} Math. Comp. 70 _(2001), no. _233,

419-430.

[3] G.P. _DRESDEN, Orbits _{of algebraic} numbers with low _heights. Math. Comp. 67 _(1998), 815-820.

[4] V. FLAMMANG, Mesures de _{polynômes. Applications} au diamètre _transfini entier. Thèse de l’Université de _Metz, 1994.

[5] V. _FLAMMANG, Two new _points in the spectrum of the absolute Mahler measure _{of totally} positive algebraic integers. Math. _Comp. 65 _(1996), 307-311.

[6] M.J. _MOSSINGHOFF, C.G. _PINNER, J.D. VAALER, Perturbing polynomials with all their roots

on the unit circle. Math. _Comp. 67 _(1998), 1707-1726.

[7] C.J. _SMYTH, On the _{product of the conjugates} outside the unit circle _of an _{algebraic integer.}

Bull. London Math. Soc. 3 _(1971), 169-175.

[8] C.J. _SMYTH, On the measure _{of totally} real _{algebraic integers} II. Math. Comp. 37 _(1981), 205-208.

[9] D. _{ZAGIER, Algebraic} numbers close both to 0 and 1. Math. Comp. 61 _(1993), 485-491. [10] S. ZHANG, Positive line bundles on arithmetic surfaces. Ann. of Math. 136 _(1992), 569-587.

Christophe DOCHE A2X Universitd Bordeaux 1 351, cours de la Liberation 33405 Talence Cedex France E-mail : cdocheGmath.u-bordeaux.fr